Weekend Diversion: The Logic That Stumped Brooklyn Nine Nine

The most famous logic puzzle from the best police comedy on television, and how to (finally) solve it!

“I have made the most important discovery of my career, the most important discovery of my life: It is only in the mysterious equations of love that any logic or reason can be found.” –John Forbes Nash, Jr.

When it comes to the power of deduction, logic is arguably the most powerful tool out there for problem-solving. By starting only with the rules of the system, it’s often possible to deduce your way to the solution without applying anything other than pure thought. Have a listen to The Logical Song by Supertramp,

while you consider the riddle posed by the police/comedy equivalent of Star Trek’s Mr. Spock, Andre Braugher’s Captain Holt:

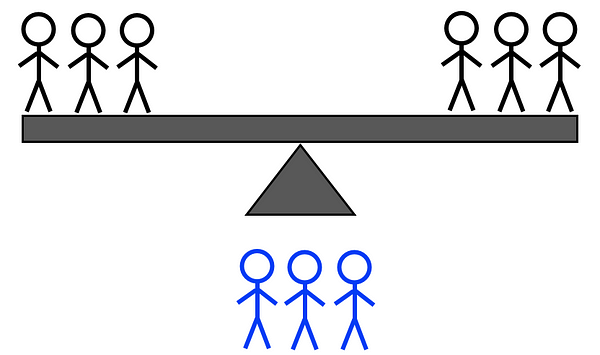
“There are 12 men on an island. 11 weigh exactly the same amount, but one of them is slightly lighter or heavier. You must figure out which. The island has no escapes, but there is a see-saw. The exciting catch? You can only use it three times.”

Image credit: E. Siegel, of the setup and starting materials.

So here’s the deal: you’ve got 12 people, 11 weigh the same, one is either heavier or lighter, and you need to figure out both which one is of unequal weight and whether they’re heavier or lighter than the rest, and you only get three uses of the see-saw to do it. It wasn’t enough to find a solution, so I went and found all possible solutions. Without further ado, here’s how you solve it.

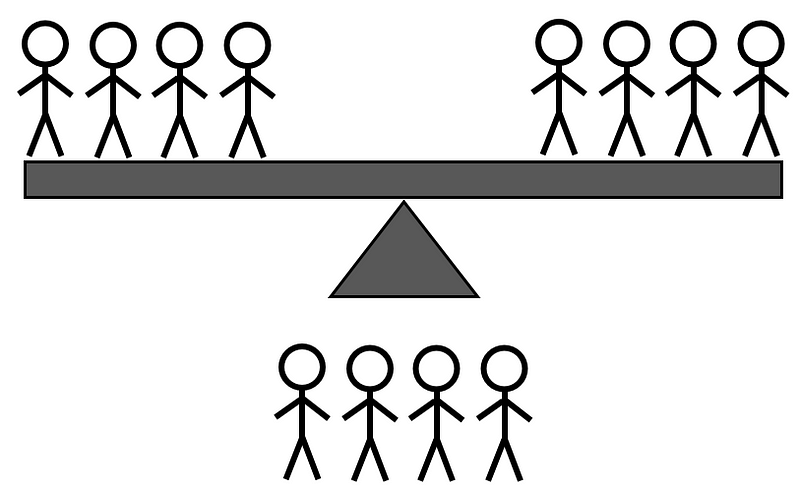
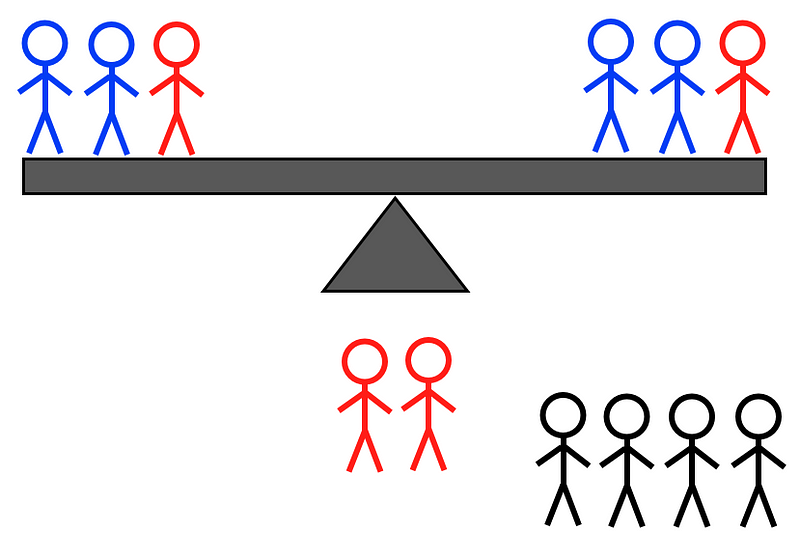
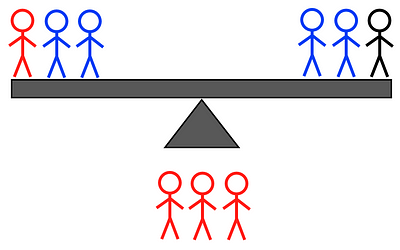
Image credit: E. Siegel, of see-saw use #1.

Weighing #1: pick eight islanders and weigh them four vs. four on either side of the see-saw. This is the only possible first step that will definitely get you to a solution. If you weigh any other combination, you lose with only two more weighings at your disposal. Here’s how the other combinations fail:

If you weigh an uneven number, you won’t have enough information.
If you weigh five vs. five or more, you won’t be able to determine both who the unequal weight person is and whether they’re lighter or heavier in the case that the scale doesn’t balance.
And if you weigh three vs. three or fewer, then if the scale balances, you won’t be able to necessarily determine both the unequal weight person’s identity and whether they’re heavier or lighter. Six or more in the final group is too many.

So, you must do four vs. four in the first step, and you’ve got two outcomes:

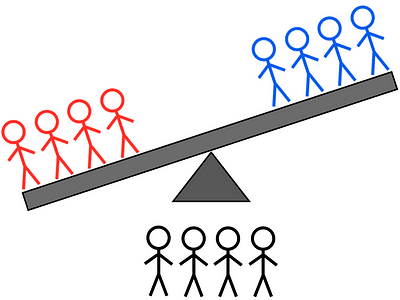
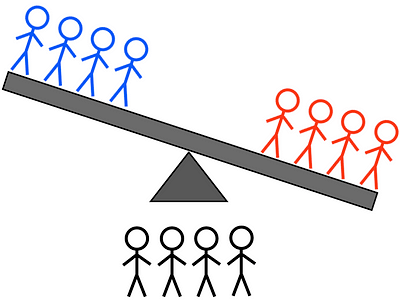
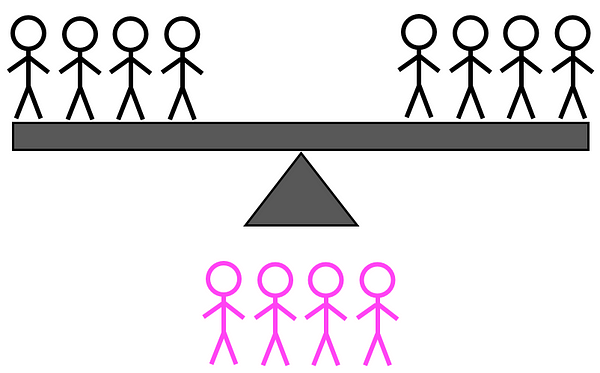
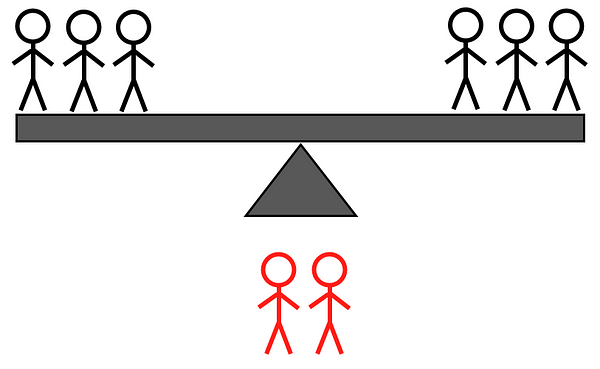
the scale tips in one direction or the other, teaching you that someone in the “heavy” group of four is heavier or someone in the “light” group of four is lighter, or the scale balances, teaching you that someone in the unweighed group of four is either heavier or lighter. Then, it’s onto the second weighing, but how you weigh depends on the outcome. First, consider the case where the first group balanced, and what we’ll need to do to that group of four to figure out who the oddly-weighted islander is, and whether they’re heavy or light.

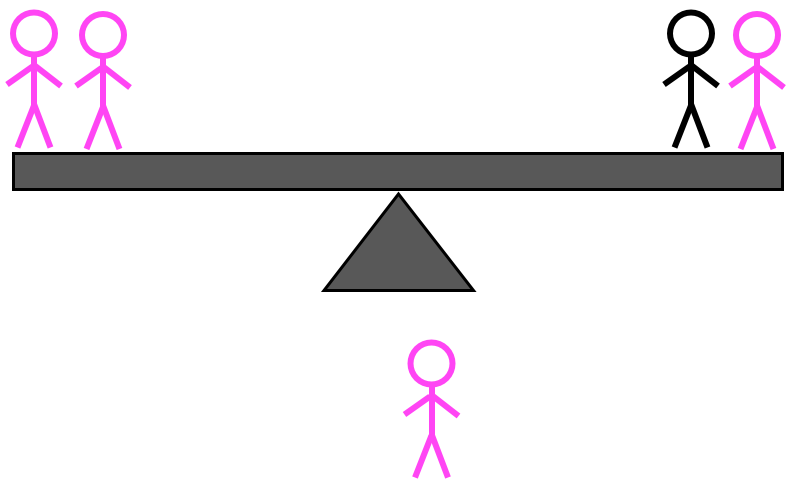
Weighing #2a: if the scale balanced during the first weighing, pick three of the unweighed islanders and one of the weighed (normal weight) islanders, and weigh them two vs. two. This is going to have three possible outcomes, each of which will teach you enough information to solve the riddle with one more weighing:

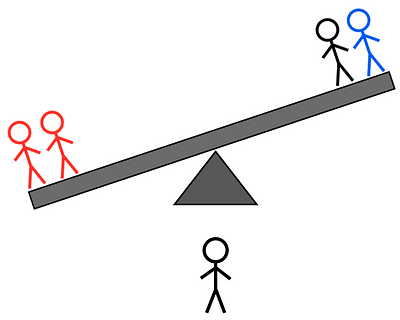
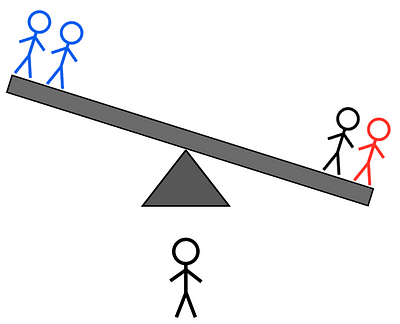
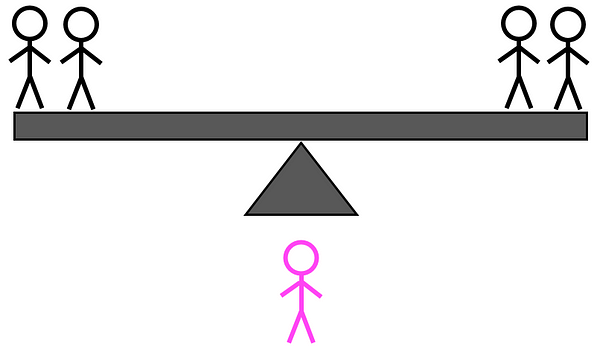
If the scale tips towards the side with two unknown islanders, either one of them is heavy or the unknown islander on the other side is light.
If the scale tips towards the side with one unknown islander, either that one is heavy or one of the two on the other side is light.
And if the scale balances, than the doubly unweighed islander is either heavy or light.

We’re so close to closing out this situation, so let’s just finish it in all three possible ways.

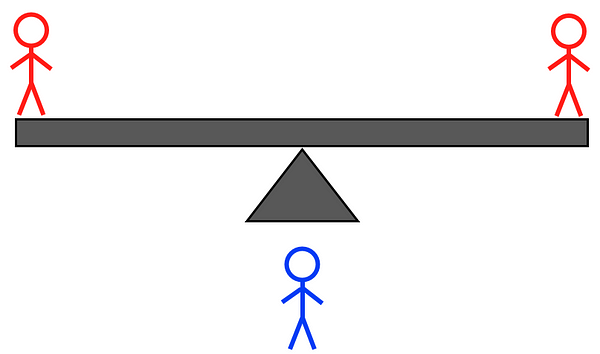
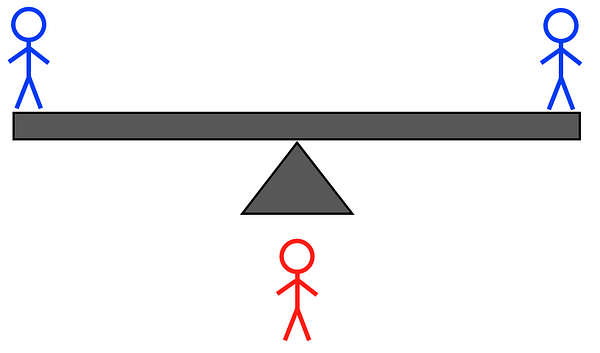
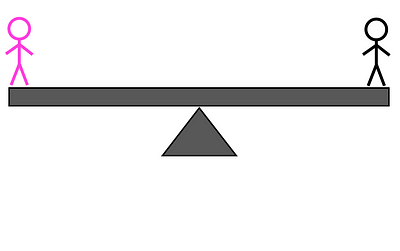
Weighing #3a: if the scale tipped in the previous weighing, weigh the two islanders who were on the doubly unknown side against one another, one vs. one; if the scale didn’t tip, weigh the doubly unweighed islander against any other islander. This will tell you both which islander is the oddly-weighted one and whether they’re heavier or lighter. Here’s the full breakdown:

If the side with two islanders weighed heavy in the second weigh-in, the see-saw can either be unbalanced or balanced. If unbalanced, the heavier side is the oddly-weighted person, and that person is heavier. If it balances, the unknown islander from the light side on the second weigh-in is the oddly-weighted person, and light.

If the side with two islanders weighed light at the second weigh-in, the see-saw will again either balance or unbalance. If unbalanced, the lighter side has the oddly-weighted person, and that person is lighter. If it balances, though, the unknown islander from the heavy side at the second weigh-in is the oddly-weighted person, and heavy.

And finally, if the second weigh-in balanced, you know who the oddly-weighted islander is. Weigh that person against anyone else, and whether that islander is “light” or “heavy” tells you that last piece of information.

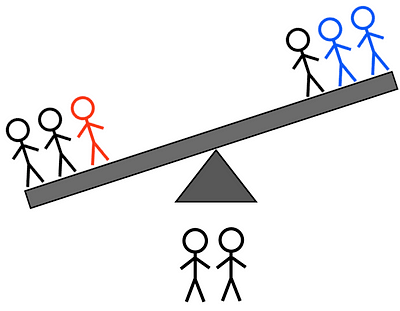
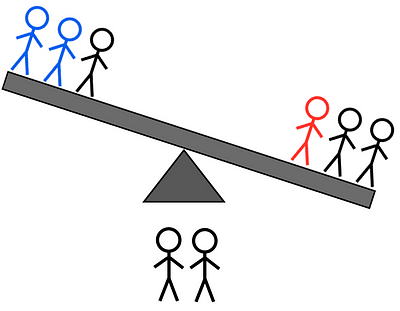
So that’s how to solve it if your initial four-on-four weighing balanced. But what if it didn’t balance? You know that either someone in a group of four is heavy or someone in another group of four is light, but that’s it. You’ve actually got a few options to lead you to the solution, but they all have some things in common. Here’s what you do next.

Image credit: E. Siegel, of one of six possible weigh-in permutations to arrive at the solution.

Weighing #2b: this needs to be a three vs. three weigh-in, and it needs to involve a total of five or six unknown-weighted islanders. It could involve all four people from one side — split two vs. two — and one or two people from the other side, either split one vs. one (if two) or (if one) weighed against a known-to-be-average-weight islander; or three from one side, split two vs. one, and two from the from the other, split one vs. one, with an average-weighted islander added to make it three vs. three. It doesn’t matter whether you weigh:

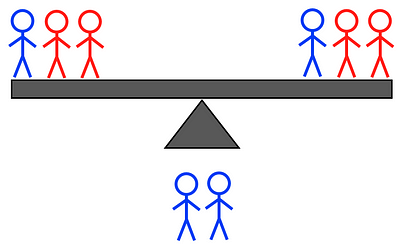
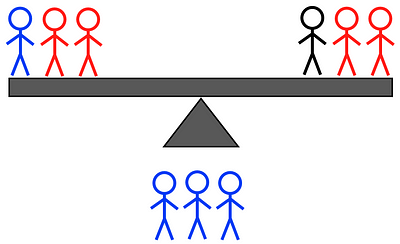
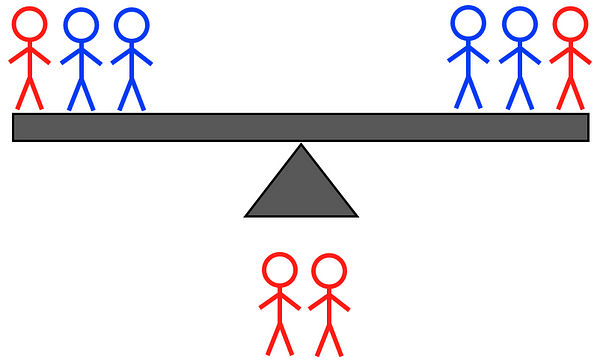
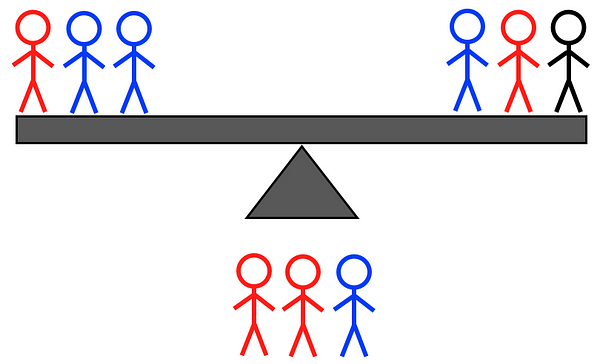
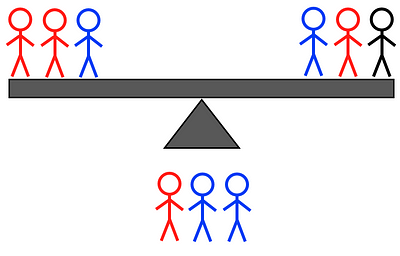
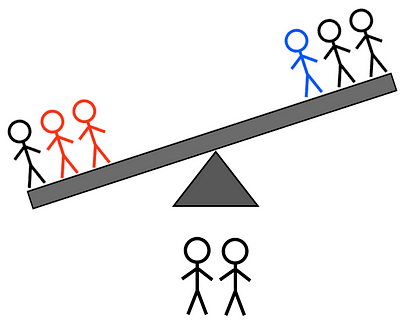
Images credit: E. Siegel, of the six scenarios for the second weigh-in if the scales didn’t balance on the first weigh-in.

two heavy and one light vs. two heavy and one light,
two heavy and one light vs. two heavy and one average,
two light and one heavy vs. two light and one heavy,
two light and one heavy vs. two light and one average,
two light and one heavy vs. one light, one heavy and one average, or
two heavy and one light vs. one light, one heavy and one average.

All six of these will bring you to the solution. The key is that either the see-saw will tip, and you’ll have only two-to-three options of who the oddly weighted person is (and you’ll already know whether they’re heavy or light), or the see-saw won’t tip, and you’ll only have two-to-three options of who the oddly-weighted person is, still already knowing whether they’re heavy or light. For comprehensiveness, let’s go through all six scenarios:

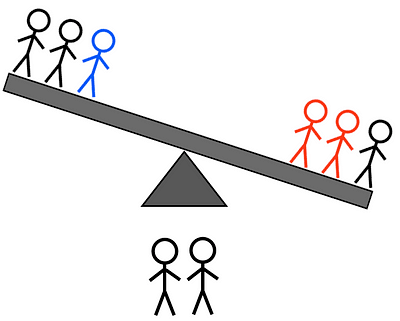
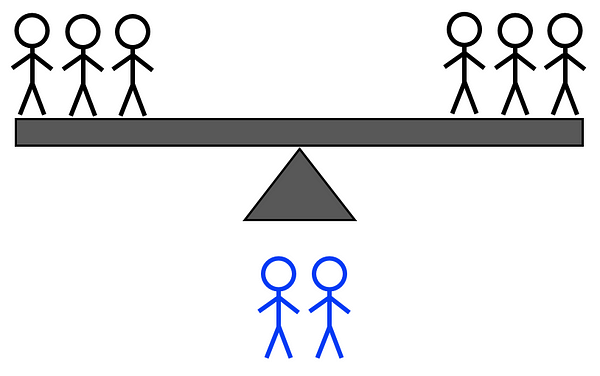
2H + 1L vs. 2H + 1L: if it tips left, you know it’s either one of the left heavies or the right light; if it tips right, you know it’s either one of the right heavies or the left light; if it doesn’t tip, you know it’s one of the two unweighed lights.

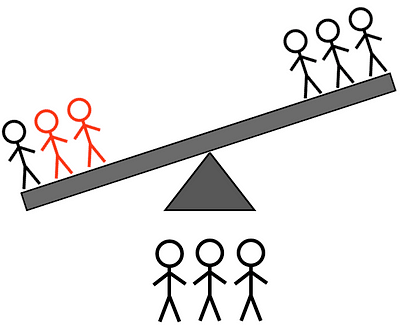
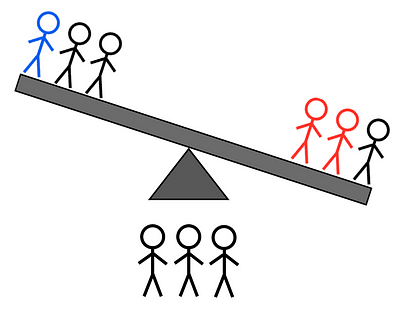
2H + 1L vs. 2H + 1A: if it tips left, you know it’s either one of the left heavies; if it tips right, you know it’s either one of the right heavies or the left light; if it doesn’t tip, it’s one of the three unweighed lights.

2L + 1H vs. 2L + 1H: if it tips left, you know it’s either the left heavy or one of the two right lights; if it tips right, you know it’s either the right heavy or one of the two left lights; if it doesn’t tip, it’s one of the two unweighed heavies.

2L + 1H vs. 2L + 1A: if it tips left, you know it’s either the left heavy or one of the two right lights; if it tips right, you know it’s one of the two left lights; if it doesn’t tip, it’s one of the three unweighed heavies.

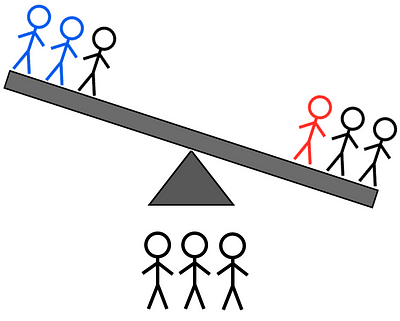
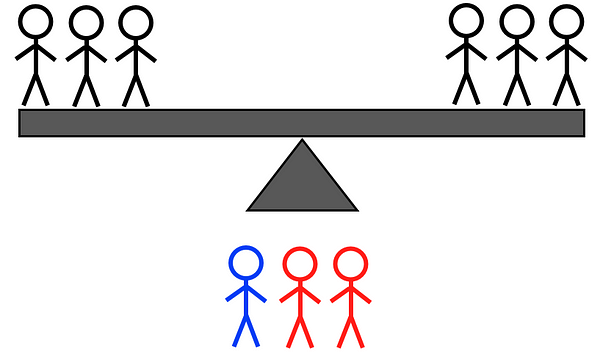
2L + 1H vs. 1L + 1H + 1A: if it tips left, it’s either the left heavy or the right light; if it tips right, it’s either the right heavy or one of the two left lights; if it doesn’t tip, it’s either the unweighed light or one of the two unweighed heavies. Or, finally,

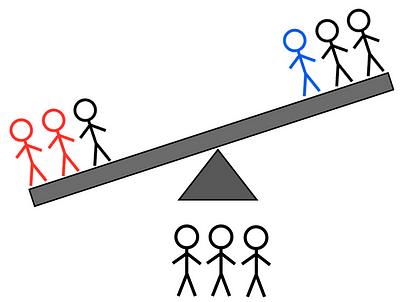
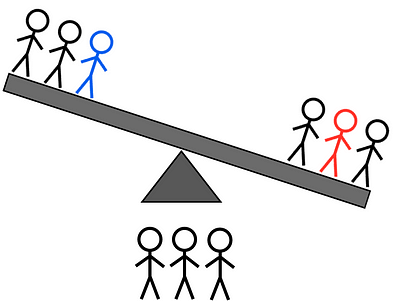
2H + 1L vs. 1L + 1H + 1A: if it tips left, it’s one of the two left heavies or the right light; if it tips right, it’s either the right heavy or the left light; if it doesn’t tip, it’s either the unweighed heavy or one of the two unweighed lights.

Notice how — after all of these situations — you’ve got it narrowed down to three possible candidates: either one of two heavies, one of three heavies, one of two lights, one of three lights, a heavy or a light, two heavies or a light, or a light and two heavies.

At this point, you have one weigh-in left, and there are only two possible things you need to do to arrive at a solution.

Image credit: E. Siegel, on the third weigh-in, when you’ve got it narrowed down to three or fewer options of known colors.

Weighing #3b: if you’ve got two+ heavies or two+ lights, weigh (two of) them one vs. one on the final see-saw; if you only have one heavy and one light, pick one and weigh them against an average-weight person. That’s it! If the see-saw balances, it’s got to be the sole person who wasn’t on the see-saw who’s the oddly-weighted individual, and you already know whether they’re lighter or heavier from the first weighing. If the see-saw doesn’t balance, you know who it is because you know whether they’re light or heavy already.

And that’s it!

If you were able to solve it, you were able to do what Captain Holt, Amy Santiago, Terry Jeffords and the rest of the Nine-Nine couldn’t do: avoid a tragic #nerdfail.

You can, by the way, always add two known, equal-weight islanders to any weigh-in, one on either side, just to get more people involved or come up with an alternate solution, as you like. Now, yes, I’m aware that the show itself put out a video of Captain Holt himself announcing the solution, but the solution is both flawed and incomplete; see if you can spot it:

https://www.youtube.com/watch?v=5K2WE9z4zL4

Hope you enjoyed this logic puzzle — and its solution — and hope to see you back here this coming week for more wonders and joys of the Universe!

Missed the best from the peanut gallery last week? Check out our comments of the week, then! And leave your comments for this at our Starts With A Bang forum.