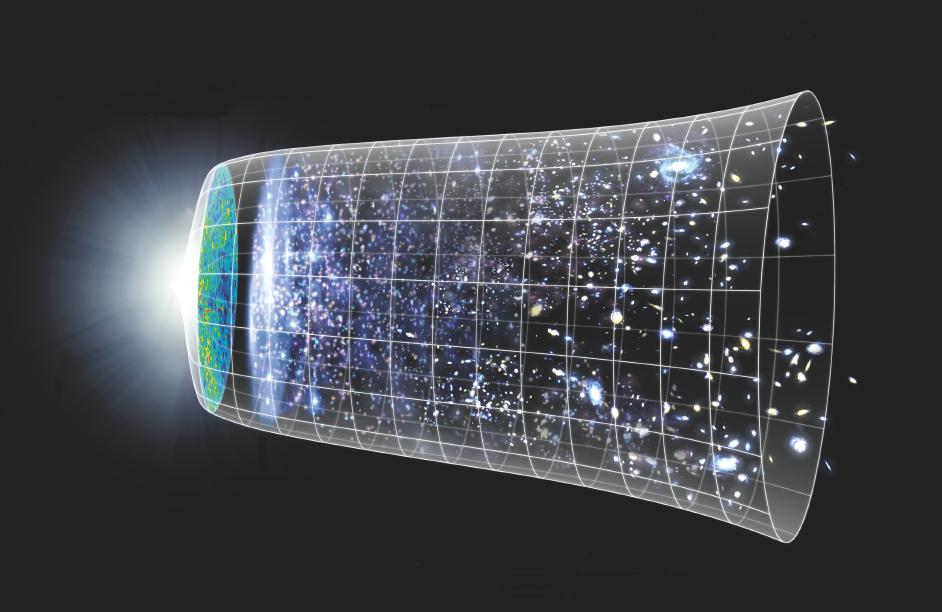
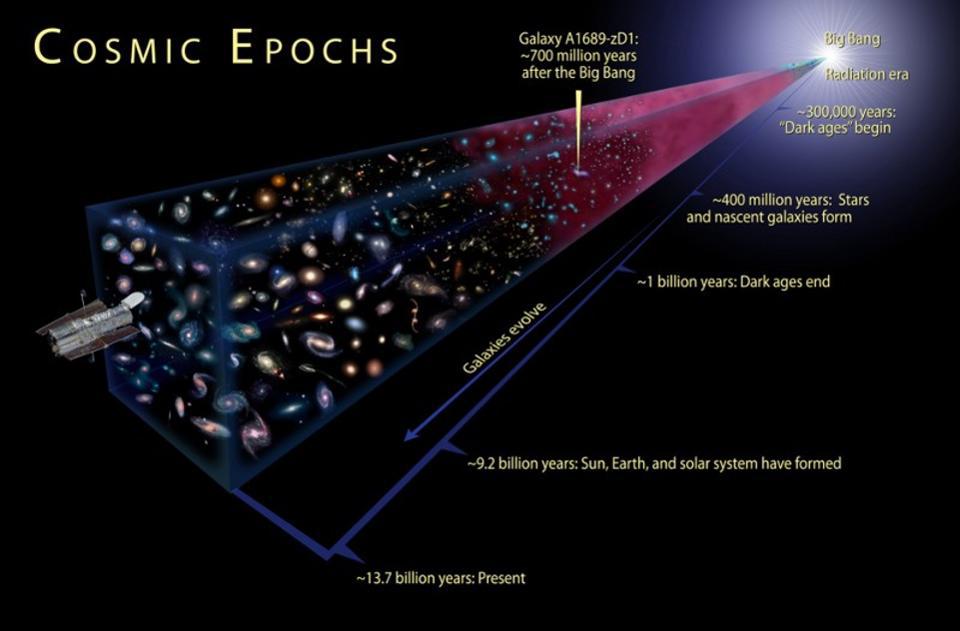
Does the expansion of the Universe break the speed of light?
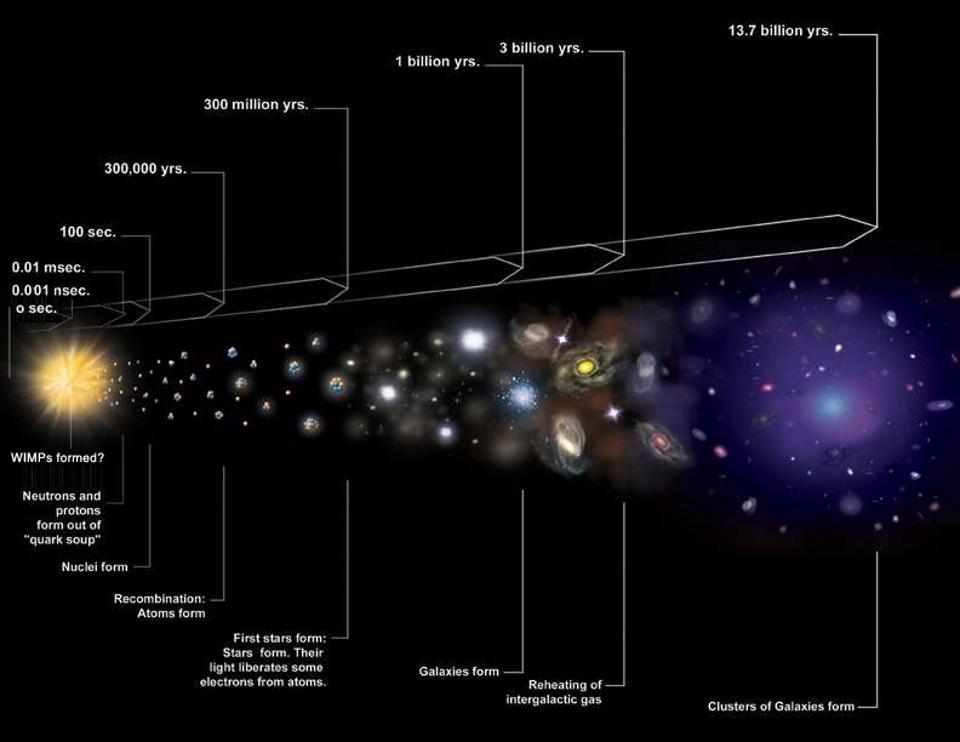
- The cardinal rule of relativity is that there’s a speed limit to the Universe, the speed of light, that nothing can break.
- And yet, when we look at the most distant of objects, their light has been traveling for no more than 13.8 billion years, but appears much farther away.
- Here’s how that doesn’t break the speed of light; it only breaks our outdated, intuitive notions of how reality ought to behave.
If there’s one rule that most people know about the Universe, it’s that there’s an ultimate speed limit that nothing can exceed: the speed of light in a vacuum. If you’re a massive particle, not only can’t you exceed that speed, but you’ll never reach it; you can only approach the speed of light. If you’re massless, you have no choice; you can only move at one speed through spacetime: the speed of light if you’re in a vacuum, or some slower speed if you’re in a medium. The faster your motion through space, the slower your motion through time, and vice versa. There’s no way around these facts, as they’re the fundamental principle on which relativity is based.
And yet, when we look out at distant objects in the Universe, they seem to defy our common-sense approach to logic. Through a series of precise observations, we’re confident that the Universe is precisely 13.8 billion years old. The most distant galaxy we’ve seen so far is presently 32 billion light-years away; the most distant light we see corresponds to a point presently 46.1 billion light-years away; and galaxies beyond about 18 billion light-years away can never be reached by us, even if we sent a signal at the speed of light today.
Still, none of this breaks the speed of light or the laws of relativity; it only breaks our intuitive notions of how things ought to behave. Here’s what everyone should know about the expanding Universe and the speed of light.

(Credit: Christopher Vitale of Networkologies and the Pratt Institute)
What “nothing can travel faster than the speed of light” actually means
It is true: Nothing can travel faster than the speed of light. But what does that actually mean? Most people, when they hear it, think the following thoughts:
- When I observe an object, I can track its motion, observing how its position changes over time.
- When I see it, I can record its observed position and the time at which I observe it.
- Then, by using the definition of velocity — that it’s a change in distance divided by a change in time — I can get its velocity.
- Therefore, whether looking at a massive or massless object, I had better observe that the velocity I get never exceeds the speed of light, or that would violate the laws of relativity.
This is true in most of our common experience, but it isn’t true universally. In particular, all of this includes an assumption that we pretty much never think about, much less state.
The assumption in question? That space is flat, uncurved, and unchanging. This occurs in Euclidean space: the type of space we normally conceive of when we think about our three-dimensional Universe. Most of us envision doing something like putting down a three-dimensional “grid” on top of everything we see and trying to describe positions and times with a set of four coordinates, one for each of the x, y, z, and time dimensions.

(Credit: Larry McNish/RASC Calgary)
In other words, most of us understand the basic concept of special relativity — the “nothing can move faster than light” part — but fail to appreciate that the real Universe cannot accurately be described by special relativity alone. Instead, we need to take into account that the Universe has a dynamical fabric of spacetime underpinning it, and that it’s only the motion of objects through that spacetime which obey those laws of special relativity.
What isn’t encapsulated in our common conception is the ways that the fabric of space departs from this idealized, flat, and three-dimensional grid, where each successive moment is described by a universally applicable clock. Instead, we have to recognize that our Universe obeys the rules of Einstein’s General Relativity, and that those rules dictate how spacetime evolves. In particular:
- space itself can either expand or contract
- space itself can be either positively or negatively curved, not only flat
- the laws of relativity apply to objects as they move through space, not to space itself
In other words, when we say “nothing can move faster than light,” we mean “nothing can move faster than light through space,” but that the motion of objects through space tells us nothing about how space itself will evolve. Alternatively, we can only assert that nothing moves faster than light relative to another object at the same location, or event, in spacetime.

Credit: E. Hubble; R. Kirshner, PNAS, 2004
Space doesn’t expand at a speed
So, nothing can move faster than light through space, but what about the ways that space itself changes? You’ve likely heard that we live in an expanding Universe, and that we’ve measured the rate at which the fabric of space itself expands: the Hubble constant. We’ve even measured that rate precisely well, and can be certain, from all the measurements and observations we’ve taken, that the present-day rate of expansion is precisely between 66 and 74 km/s/Mpc: kilometers-per-second-per-megaparsec.
But what does it mean that space is expanding?
For every megaparsec (about 3.26 million light-years) away that a distant and unbound object is from us, we’ll see it recede from us as though it were moving away at the equivalent of 66-74 km/s. If something is 20 Mpc away from us, we’d expect to see it moving away at the equivalent of 1320-1480 km/s from us; if it’s 5000 Mpc away, we’d expect to see it moving away at ~330,000-370,000 km/s.
But this is confusing for two reasons. One, it’s not actually moving at that speed through space, but rather this is the effect of the space between objects expanding. And two, the speed of light is 299,792 km/s, so isn’t that hypothetical object that’s ~5000 Mpc away actually moving away from us at speeds exceeding the speed of light?
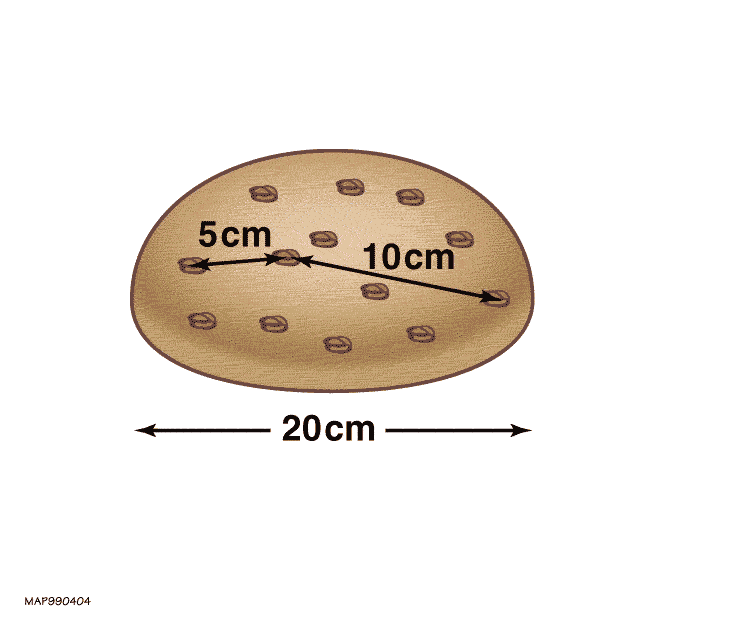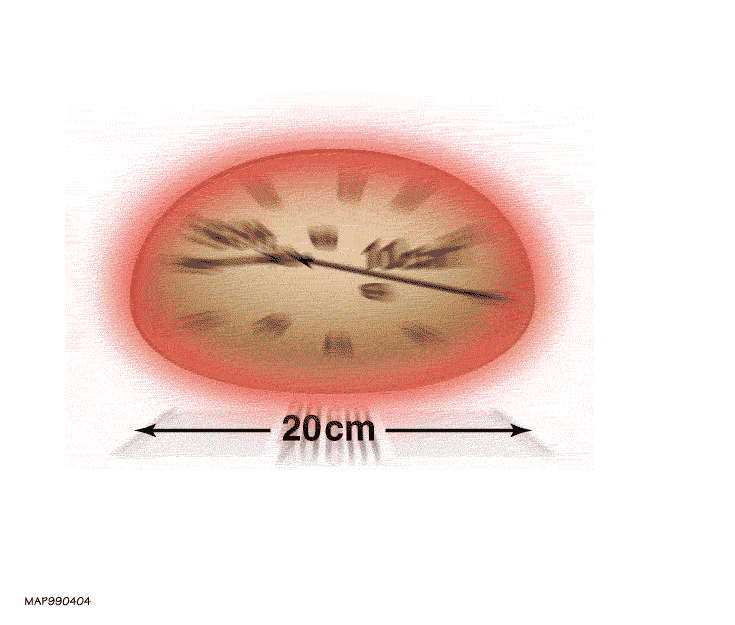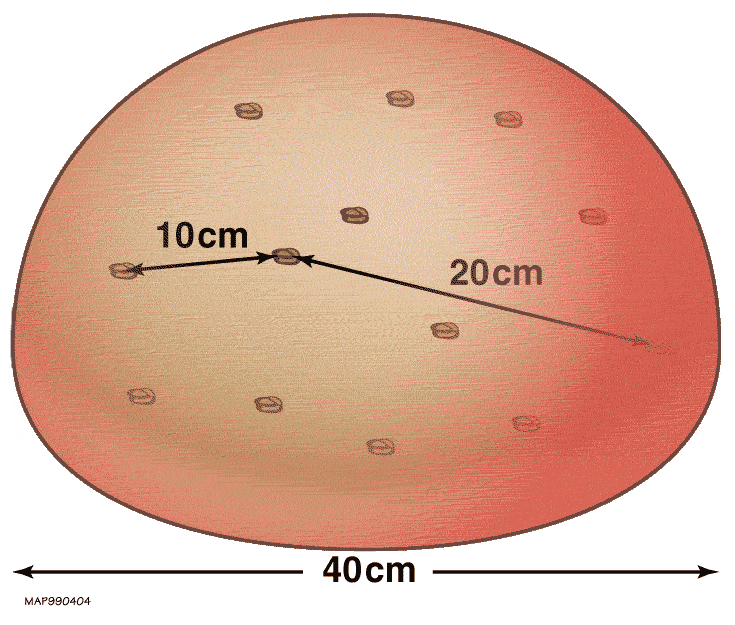
The way I like to think about the expanding Universe is with the “raisin bread” model. Imagine that you have a ball of dough with raisins all throughout it. Now imagine that the dough leavens, expanding in all directions. (If you like, you can further imagine this is happening in a zero-gravity environment, like on the International Space Station.) Now, if you put your finger down on one raisin, what do you see the other raisins doing?
- The closest raisins to you will appear to move slowly away from you, as the dough between them expands.
- Raisins that are farther away will appear to be moving away more quickly, as there’s more dough between them and you than the closer raisins.
- Raisins that are even farther away will appear to be moving away ever more-and-more quickly.
Now, in our analogy here, the raisins are like galaxies or bound groups/clusters of galaxies, and the dough is like the expanding Universe. But in this case, the dough that represents the fabric of space cannot be seen or directly detected, doesn’t actually get less dense as the Universe expands, and simply provides a “stage” for the raisins, or galaxies, to inhabit.

Credit: E. Siegel/Beyond the Galaxy
The rate of expansion is dependent on the total amount of “stuff” in a given volume of space, so as the Universe expands, it dilutes and the expansion rate drops. Because matter and radiation are made up of a fixed number of particles, as the Universe expands and the volume increases, the density of matter and radiation both drop. The density of radiation drops a little faster than the density of matter, because radiation’s energy is defined by its wavelength, and as the Universe expands, that wavelength stretches as well, causing it to lose energy.
On the other hand, the “dough” itself contains a finite, positive, non-zero amount of energy in every region of space, and as the Universe expands, that energy density remains constant. While the matter and radiation densities drop, the energy of the “dough” (or space) itself remains constant, and that’s what we observe as dark energy. In our real Universe, which contains all three of these, we can confidently conclude that the Universe’s energy budget was dominated by radiation for the first few thousand years, then by matter for the next few billion years, and then by dark energy thereafter. As far as we can tell, dark energy will continue to dominate the Universe forever.
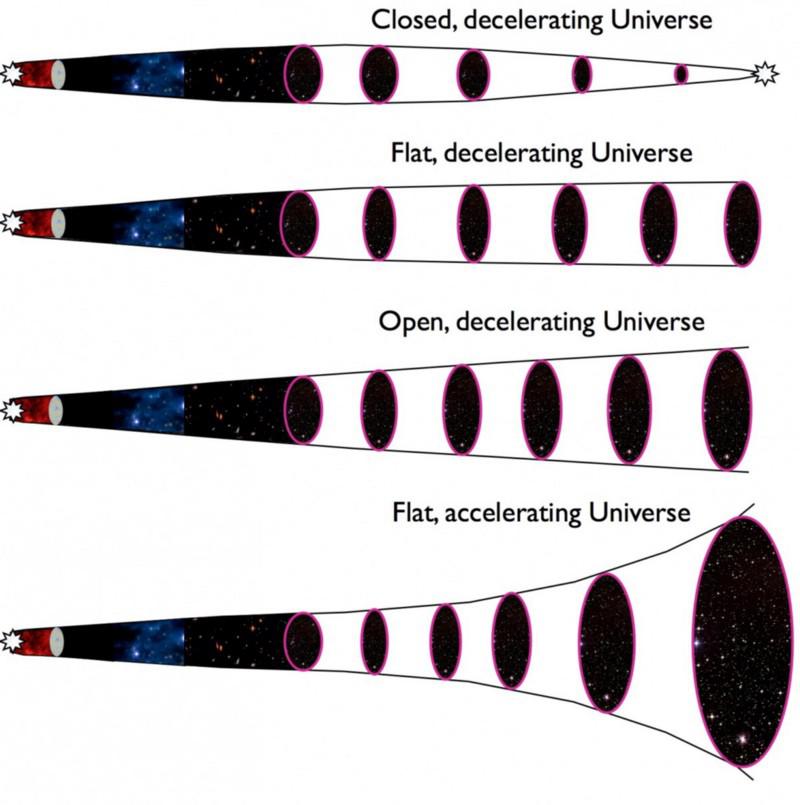
(Credit: E. Siegel/Beyond the Galaxy)
Now, here’s the tricky part. Every time we look at a distant galaxy, we’re seeing the light from it as it is right now: upon its arrival. That means the light that was emitted experiences a slew of combined effects:
- the difference between the gravitational potential from where it was emitted to where it arrives
- the difference in the motion of the emitting object through its space and the motion of the absorbing object through its local space
- the cumulative effects of the expansion of the Universe, which stretch the light’s wavelength
The first part, thankfully, is normally very small. The second part is known as peculiar velocity, which can range from hundreds up to a few thousand kilometers-per-second.
Credit: Rob Knop
But the third part is the effect of cosmic expansion. At distances of more than about ~100 megaparsecs or so, it’s always the dominant effect. On the largest cosmic scales, the expansion of the Universe is all that matters. What’s important to recognize is that the expansion doesn’t have an intrinsic speed to it at all; space expands at a frequency: a speed-per-unit distance. Expressing it as some amount of kilometers-per-second-per-megaparsec obscures that “kilometers” and “megaparsecs” are both distances, and they’ll cancel if you convert one into the other.
The light from distant objects does indeed get redshifted, but not because anything is receding faster than light, nor because anything is expanding faster than light. Space simply expands; it’s us who shoehorns in a “speed” because that’s what we’re familiar with.
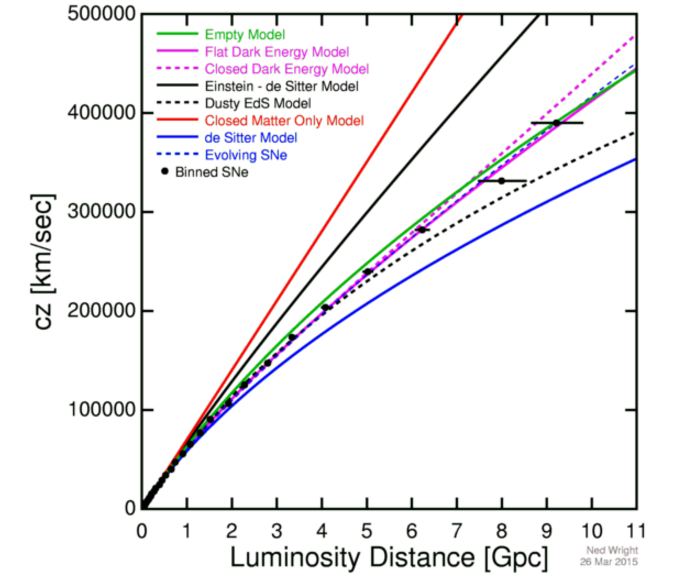
What’s actually accelerating in our accelerating Universe?
One difficulty that we have is that we can’t actually measure the speed of a distant object. We can measure its distance through a variety of proxies, like how bright/faint it is or how large/small it appears on the sky, presuming we know or can figure out how intrinsically bright or large it is. We can also measure its redshift, or how the light is “shifted” from how it would be if we were in the precise location and under the same precise conditions where the light was emitted. That shift, because of our familiarity with how waves shift due to the Doppler effect (such as for sound waves), is something we often translate into a recession speed.
However, we’re not measuring an actual speed; we’re measuring the cumulative effects of motions plus the effect of the expanding Universe. When we say “the Universe is accelerating,” what we actually mean — and this is not what you’d intuit at all — is that if you watch the same object as the Universe expands, it will not only continue to increase in distance from you, getting farther and farther away, but the light that you receive from this object will continue to display an ever-increasing redshift, which makes it seem as though it’s accelerating away from you.
In reality, though, the redshift is due to the expansion of space, not to the galaxy speeding away from you faster and faster. The expansion rate, if we were to actually measure that over time, is still decreasing, and will eventually asymptote to a finite, positive, and non-zero value; that’s what it means to live in a dark energy-dominated Universe.
(Credit: Andrew Z. Colvin and Frederic Michel, Wikimedia Commons; Annotations: E. Siegel)
So what determines “distance” in an expanding Universe?
When we talk about the distance to an object in the expanding Universe, we’re always taking a cosmic snapshot — a sort of “God’s eye view” — of how things are at this particular instant in time: when the light from these distant objects arrives. We know that we’re seeing these objects as they were in the distant past, not as they are today — some 13.8 billion years after the Big Bang — but rather as they were when they emitted the light that arrives today.
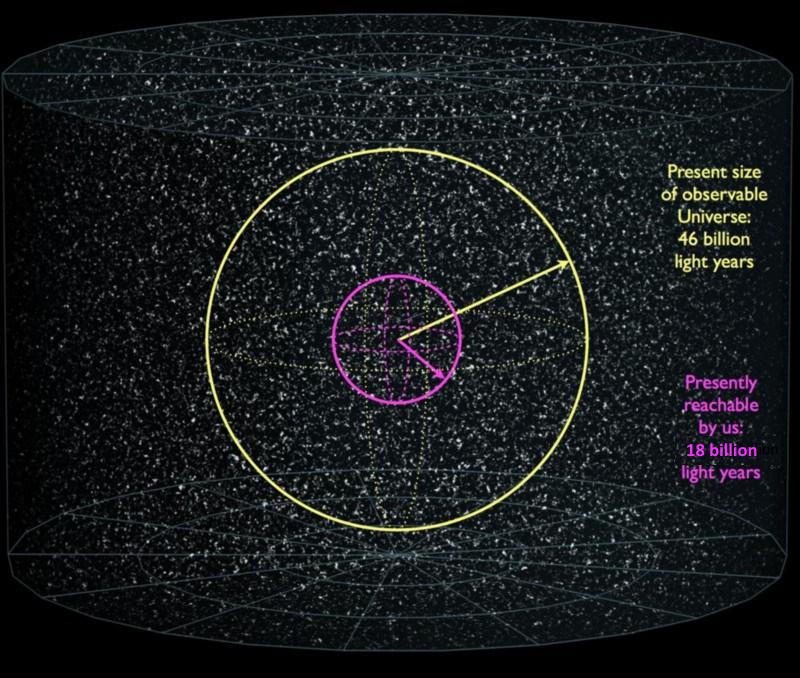
But when we talk about, “how far away is this object,” we’re not asking how far away it was from us when it emitted the light we’re now seeing, and we aren’t asking how long the light has been in transit. Instead, we’re asking how far away the object, if we could somehow “freeze” the expansion of the Universe right now, is located from us at this very instant. The farthest observed galaxy GN-z11, emitted its now-arriving light 13.4 billion years ago, and is located some 32 billion light-years away. If we could see all the way back to the instant of the Big Bang, we’d be seeing 46.1 billion light-years away, and if we wanted to know the most distant object whose light hasn’t yet reached us, but will someday, that’s presently a distance of ~61 billion light-years away: the future visibility limit.
Just because you can see it, though, doesn’t mean you can reach it. Any object presently beyond 18 billion light-years from us will still emit light, and that light will travel through the Universe, but the fabric of space will simply expand too relentlessly for it to ever reach us. With each moment that passes, every unbound object moves farther and farther away, and previously reachable objects transition across that mark to become forever unreachable. Nothing moves faster than light in an expanding Universe, and that’s both a blessing and a curse. Unless we figure out how to overcome this, all but the closest galaxies may forever be beyond our reach.