Throwback Thursday: The Physics of an Inclined Treadmill

Is running up an inclined treadmill the same, physically, as running up an actual mountain? Here’s what science has to say.

“Nothing worth having was ever achieved without effort.” -Teddy Roosevelt

One of the most popular exercises at the gym is the treadmill. And why wouldn’t it be? Whether you’re running or walking, it’s a great way to get your heart rate up, get your body moving, and for many people, a great way to burn calories.

But whether you’re walking, jogging or running, there’s one extremely simple thing you can do to dramatically intensify your workout: incline it!

If you’re an outdoor walker/runner, you might wonder whether this is really the equivalent of going uphill instead of over level ground, or whether there’s a fundamental difference between the two situations. There are a great many physiological differences between walking along an incline versus on level ground, but what does physics have to say about the inclined treadmill versus an actual uphill journey?

Let’s start with the basics to get a firm footing on what we’re looking at: if you’re on level ground (or a level treadmill), you stay at the same level in the Earth’s gravitational field. With every step you take, gravity is pulling you towards the center of the Earth, and while your feet have to exert an equal-and-opposite force upwards to keep you from sinking, there is no up-or-down motion; you only move horizontally, or perpendicular to the gravitational force.

In physics terms, that means you’re not doing any work. You’re working hard, of course, and exerting yourself tremendously, but unless you’re propelling yourself over a distance in the same direction as a force — in this case, the force of gravity — you’re not doing any physical work.

But if you walk uphill, you not only need to move forward at whatever pace you were moving at, you also need to climb — a little with every step — out of the Earth’s gravitational field!

The Earth’s gravitational field is no slouch, either. I’m an 85 kg individual or so, and for me to raise my elevation by just 5 meters (about 16 feet) costs me 4,200 Joules of energy, also known as one food calorie.

Now, if I actually exercise, I burn significantly more than one calorie by raising myself those 5 meters. Why’s that? The two most significant reasons are as follows:

1. I am not a perfect engine. This means, in order for me to do 4,200 Joules of physical work, I need to burn about three times as much in food energy in order to get that much useful energy out. Alas, our bodies are inefficient in that manner.
2. When you exercise and then stop, your body doesn’t know that it’s okay for your heart to slow down for quite some time. So spending an hour walking uphill will elevate my metabolic rate for a lot longer than an hour!

Ahh, the power of exercising. But I’m not a physiologist; I deal in terms of physical work alone. So, just looking at the extra amount of energy you’d have to spend to climb up an incline rather than level ground, what are we talking about?

Let’s make a helpful table. We’ll just look at the total distance you travel (e.g., if you walk at three miles-per-hour for one hour, you go three miles), put in the incline, and see how much extra physical work you need to do!

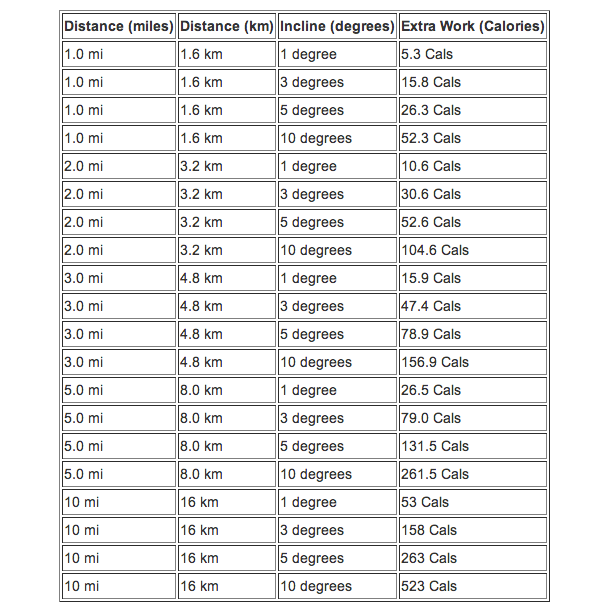
This is all for a person with a mass of 80 kg (about 176 pounds), or a little bit lighter than I am. Doesn’t the incline make a spectacular difference? In other words, if you make a long-term change from walking on a flat ground (or treadmill) to walking up inclined ground, you must burn extra energy with every step you take!

But what about the treadmill? Is walking up an inclined treadmill the same as walking up a hill? Think about it for a minute. On the one hand, you don’t rise any higher in a gravitational field when you’re on a treadmill, and in that sense, it’s a little different than walking uphill. But the different of a few hundred (or even a few thousand) meters makes very little difference in terms of the force of gravity that’s exerted on your body by the Earth.

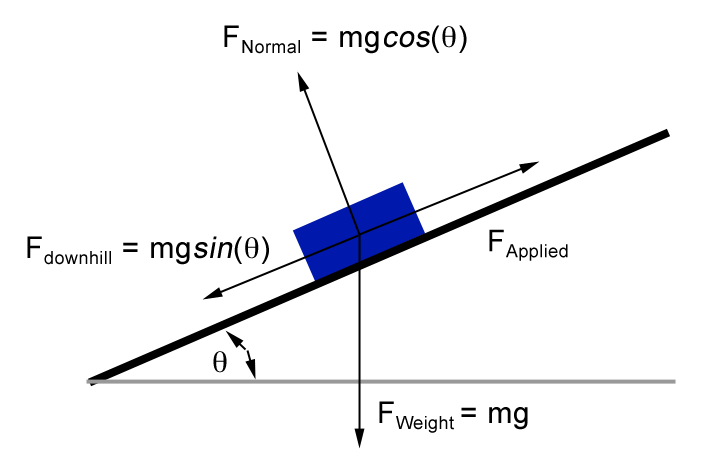
In both cases — up an actual hill and up an inclined treadmill — you need to exert a portion of your force in the direction directly opposed to the Earth’s gravitational force in order to walk along the surface, and that means you need to do work against the force of gravity!

The only difference between an inclined treadmill and a straight path along an inclined mountain is that if you don’t walk at all, you remain stationary, while on the treadmill, you get transported down as though you were on a conveyor belt.

It doesn’t matter whether the belt is stationary, moving up or moving down: if you’re ascending on it under the power of your own legs, you still have to do the work with your legs with every step you take. Every step does work against the force of gravity, and all the other particulars of the problem — including the speed of the treadmill, the direction of the conveyor, or the altitude — are irrelevant, by Einstein’s equivalence principle! So climb a mountain or climb the same incline on your treadmill, it doesn’t matter: the physical work you do will be the same!

Now if only I can explain Roger G’s moves in the Michael Jackson “Bad” video.

I suppose that’s a story for another time!

An earlier version of this post originally appeared on the old Starts With A Bang blog at Scienceblogs.