This Is Why We’ll Never Detect Hawking Radiation From An Actual Black Hole

The theoretical reasons to expect it are compelling, but the technology required to detect it is unfathomable.

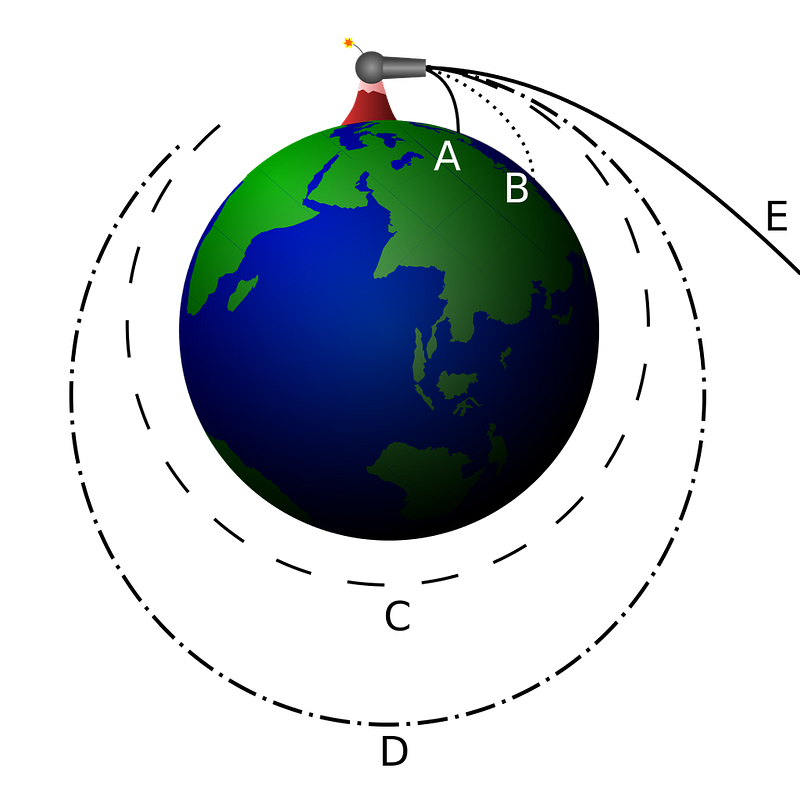
All throughout our galaxy, millions of black holes of a variety of masses orbit, subject to the same rules of gravitation as every other mass in the Universe. Only, instead of emitting light dependent on their surface area and temperature, they’re completely black. Whatever exists at the singularity that’s shrouded behind each black hole’s event horizon, we cannot see it. From within a black hole, nothing, not even light, can escape.

The only light we’ve ever observed from a black hole doesn’t come from inside the black hole itself, but rather from accelerated matter that interacts somewhere outside of the event horizon. However, there is a very particular type of light that black holes ought to emit: Hawking radiation, arguably the greatest breakthrough of Stephen Hawking’s scientific career. Unfortunately, it’s almost certain that we’ll never detect it. Here’s the science of why.

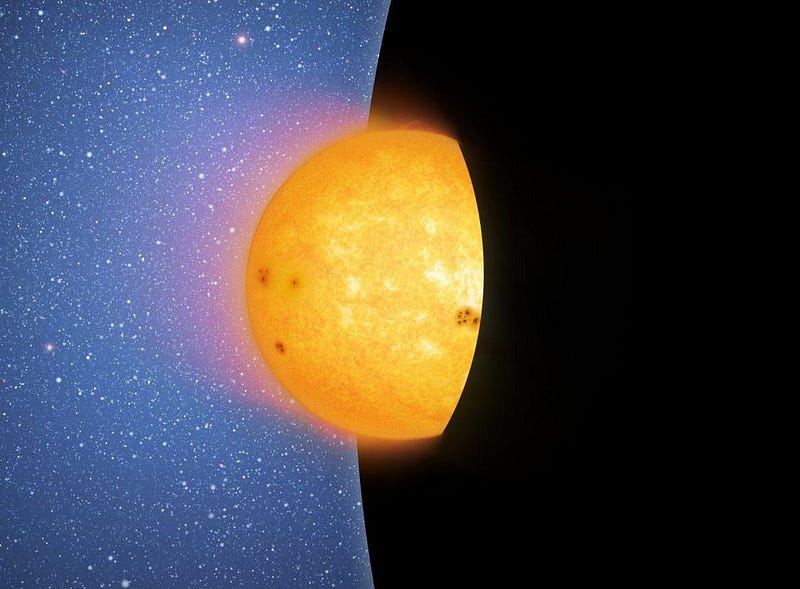
Black holes, contrary to what you might expect, are an idea that’s hundreds of years old. Way back in the 18th century, when Newtonian physics was the only game in town, scientist John Michell came up with a brilliant realization concerning the Sun. If you assumed that the Sun was a low-density sphere but imagined that there was more of it — yielding an object that was more massive and took up a greater volume — then once you crossed a critical threshold, light would be unable to escape from it.

At its current size and mass, you’d have to reach a speed of 618 km/s to escape from the Sun from its edge. Light, which travels at 300,000 km/s, can easily do that. But if you dumped enough mass into this object, its escape velocity would rise and rise. Once it exceeded 300,000 km/s, light emitted from its surface would curve back onto the object itself. You would create what we now know as a black hole.

This idea gained new life in the 20th century, after Einstein put forth his general theory of relativity, which superseded Newton’s theory of gravity. Gravity wasn’t caused by an invisible force attracting all masses in the Universe to one another dependent on the distance between them. Instead, the Universe was a fabric where space and time were their own inseparable entity — spacetime — and the presence of matter and energy curved that spacetime.

Whereas to Newton, objects always moved in straight lines unless an outside force caused them to accelerate, Einstein dictated that all objects followed the curved path laid out to them by whatever shape spacetime took. Matter and energy caused spacetime to curve, and that curved spacetime told matter how to move. In 1915, Einstein put forth the final version of General Relativity for the first time. By January of 1916, the first exact solution had been found.

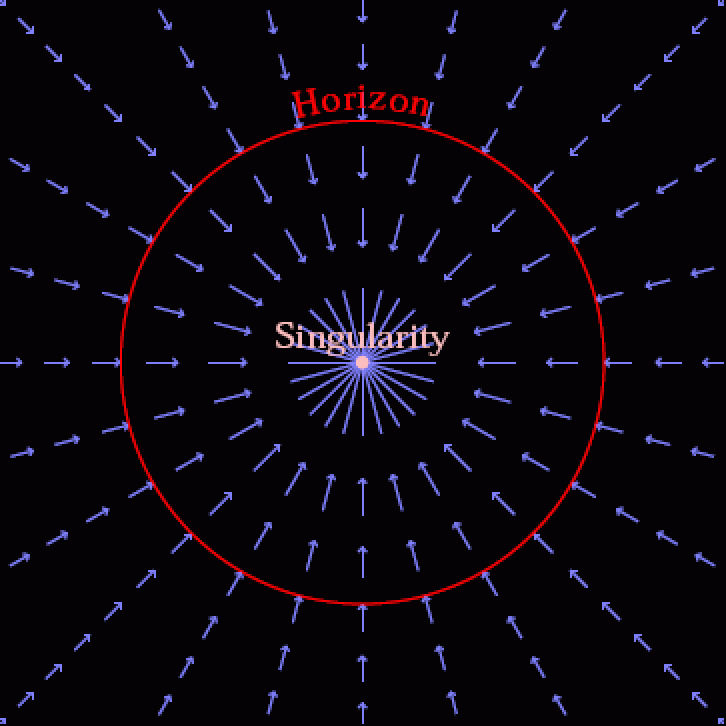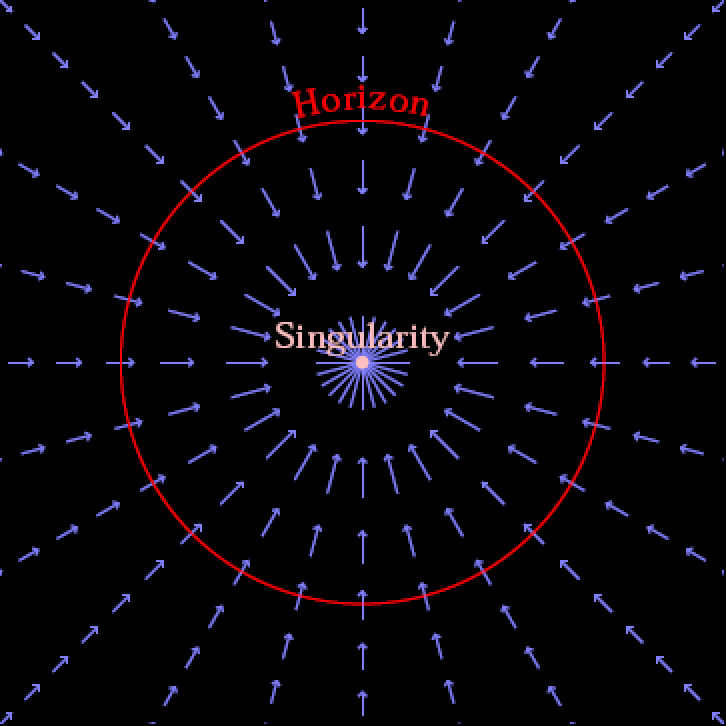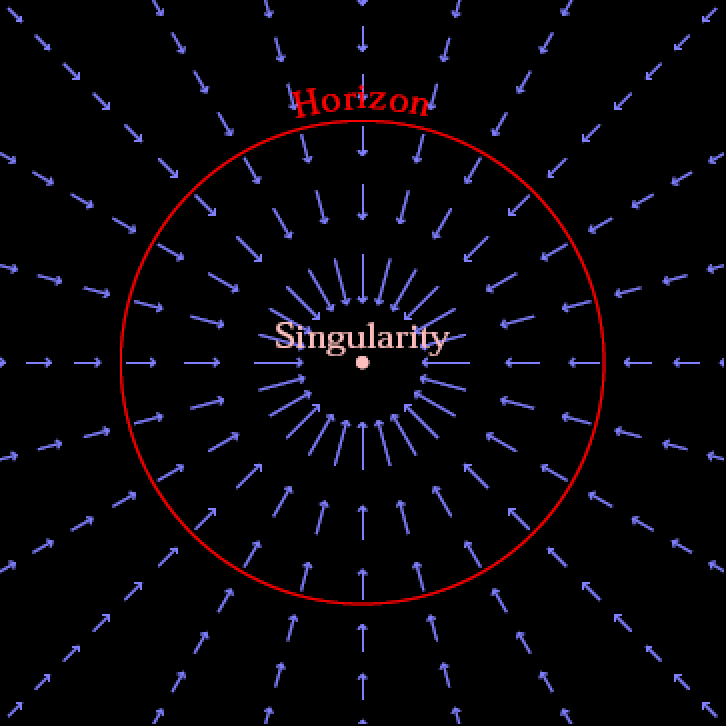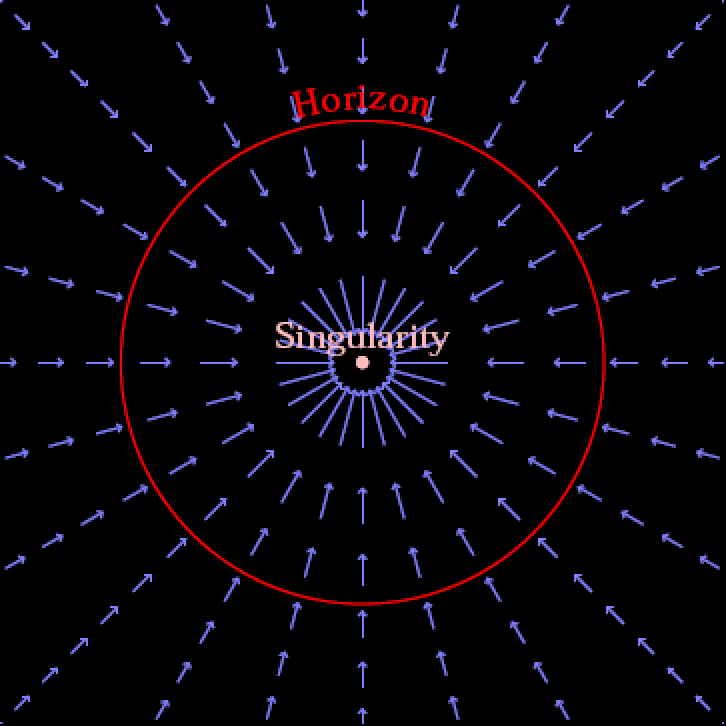
That solution was found by Karl Schwarzschild, and corresponds to what we now know as a non-rotating black hole. Initially, Schwarzschild considered a very simple system: a Universe, governed by General Relativity, with one massive “point” in it and nothing else. And yet, there’s a tremendous amount of deep physics encoded in this system, what we now call the Schwarzschild solution in the context of this field.

Yes, far away from this point mass, gravitation acts very similarly to Newton’s predictions: gravity behaves almost identically to Newton’s force law for Universal gravitation.

But close to the mass — where gravitational fields get strong — space is curved more severely, and there’s an “extra” attraction beyond what Newton predicts.

And if you get too close, you’ll encounter the event horizon: a region from which nothing, not even light, can escape.

Over the subsequent decades, additional solutions were found that extended Schwarzschild’s original work. You could have not only mass but electric charge to your point mass, leading to a Reissner-Nordström (rather than a Schwarzschild) black hole. You could add in angular momentum (i.e., rotation), leading to a (realistic) Kerr black hole. And you could have all three: mass, charge, and angular momentum, leading to a Kerr-Newman black hole.

Each one still has an event horizon, where outside of the horizon, light can escape, while interior to it, anything moving at light speed or slower cannot escape. Just outside the event horizon of each one, spacetime is curved far more significantly than Newton would have predicted. It wasn’t until the 1960s and 1970s, however, that people started realizing something very profound about the quantum implications for the regions near these event horizons.

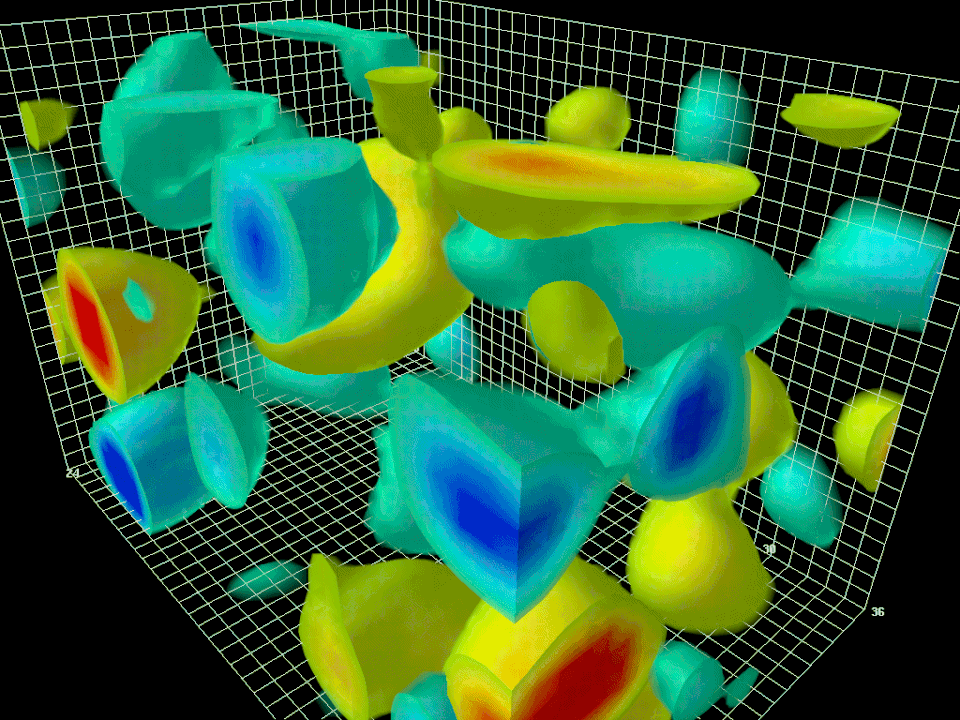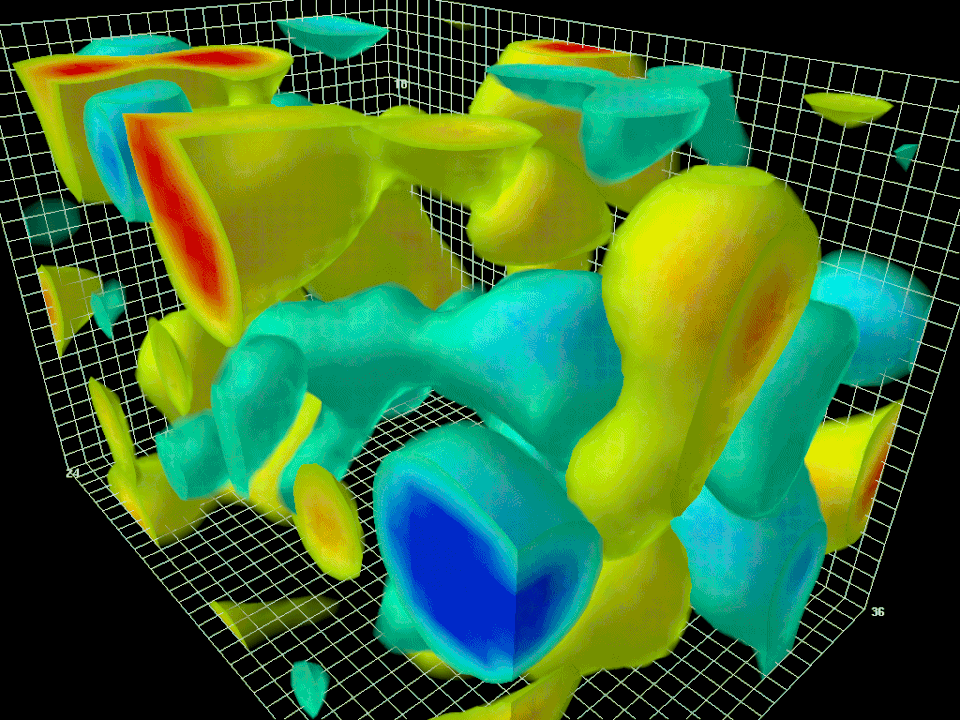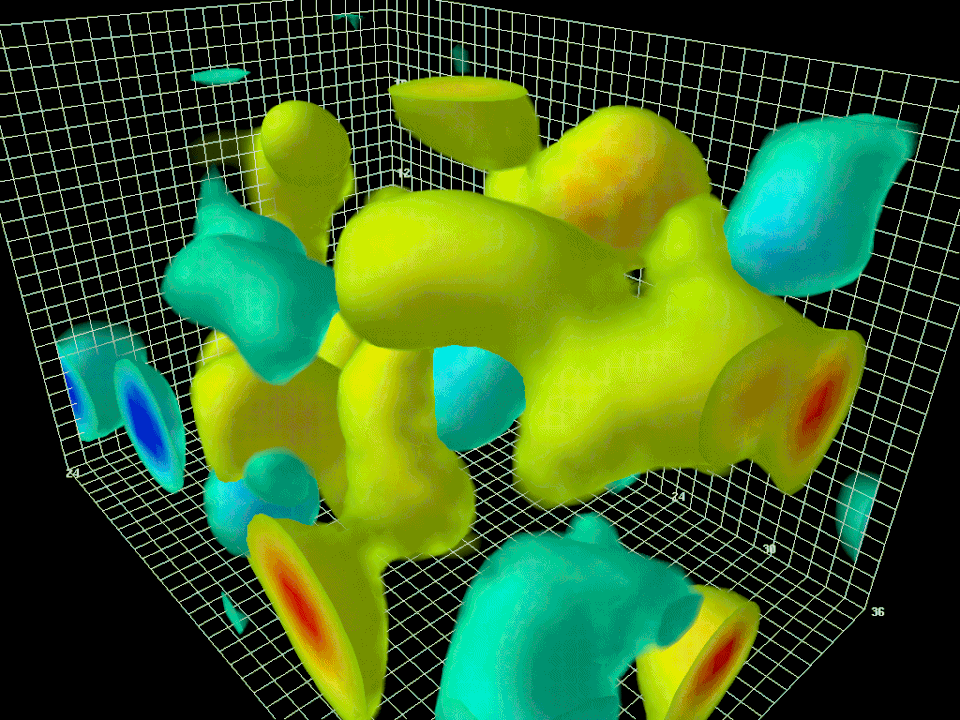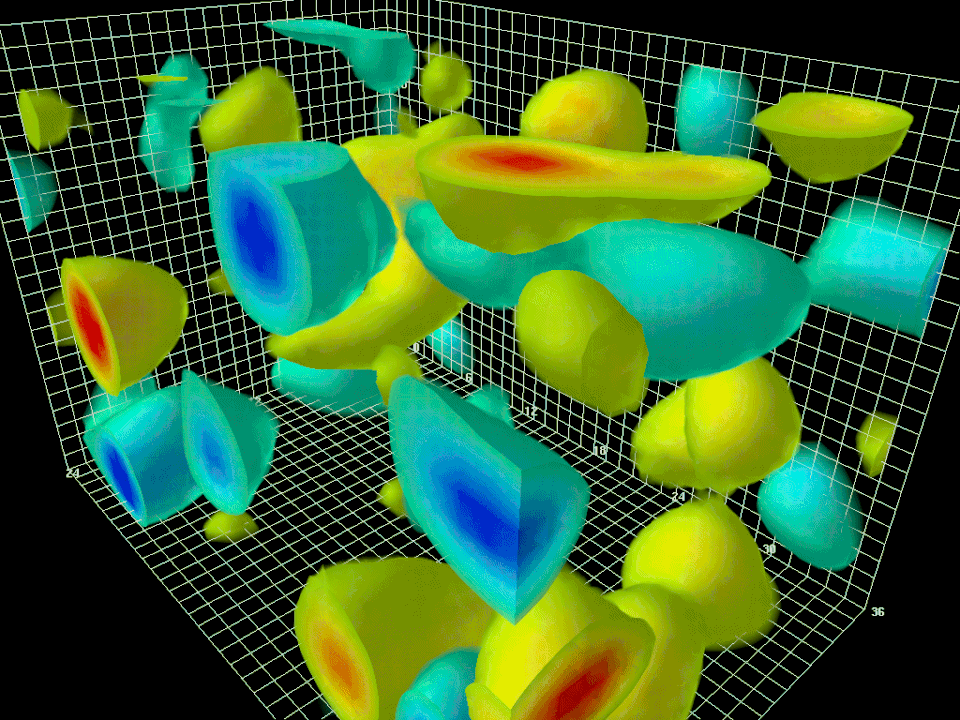
You see, in quantum field theory, empty space isn’t so empty. What we think of as empty space — space without any masses, particles, or quanta of energy in it — is only empty in a sense. Yes, there might not be individual quanta of mass or energy in them, but the quantum fields that govern the Universe are still there. They’re just in their ground state: the lowest energy state possible.

What we think of as particles correspond to excitations of the various quantum fields, and so only in the non-excited state can you have no particles at all. But even in that scenario, the fields themselves are still there. They still have an underlying energy that is not required to be zero, and they still obey Heisenberg’s uncertainty principle, which tells us that for any finite interval of time we look at, there’s a limit to the certainty with which we can know the energy of a system.

This leads us to, perhaps, the most accurate way to think about the zero-point energy of empty space itself. Space is filled with quantum fields, and even in the absence of all matter and energy, those fields have inherent fluctuations in their values at any particular time. It’s like a foamy, wavy ocean: flat from afar, churning and unstable up close. As long as you’re floating in it, however, your head will stay above water.

Now, think about what this means for flat space, far away from any masses or sources of spacetime curvature, versus for the curved space very close to the event horizon of a black hole. Yes, wherever you are, you’ll float just fine; you’ll see a similar ocean wherever you are. But someone in the curved-space ocean disagrees with someone in the flat-space ocean as far as how to keep your head above water. To move from one spot to another, you need to change your proverbial “depth” in the cosmic ocean of the quantum vacuum.

This is where Hawking radiation comes from. Observers in regions of space with different amounts of spatial curvature disagree with one another as to what the zero-point energy of the quantum vacuum is. The difference in the values of quantum fields at various points in severely curved space is what leads to the production of radiation, also explaining why the radiation is produced over a large volume surrounding the black hole, not solely at the event horizon.

The next question — which is where Hawking did his most spectacular work in 1974 — is to answer these questions: what are the temperature, flux, and energy spectrum of this Hawking radiation? The answer, quite wonderfully, is simple: the spectrum is always a blackbody, while the temperature and flux are pretty much solely determined by the mass. But, perhaps ironically, the larger the black hole’s mass, the smaller the temperature and flux.

In other words, heavier black holes emit lower-temperature and lower-energy Hawking radiation, and less of it, too. The temperature is inversely proportional to the mass, while the flux is inversely proportional to the mass squared. Put those together, and it means that more massive black holes live longer by a factor of their mass cubed. If we want to know where to go to find the brightest sources of Hawking radiation, we have to find the lowest-mass black holes of all.

Travel the Universe with astrophysicist Ethan Siegel. Subscribers will get the newsletter every Saturday. All aboard!

Unfortunately, the minimum mass for a black hole that our Universe is capable of creating is somewhere around 2.5 solar masses: heavier than even our own Sun. It would have a temperature of around 25 nanokelvin, a signal virtually impossible to disentangle against the noise provided by the Cosmic Microwave Background, some 100 million times hotter. Unless much lower mass black holes exist, and the data strongly disfavors the existence of these primordial black holes, Hawking radiation should remain undetectable.

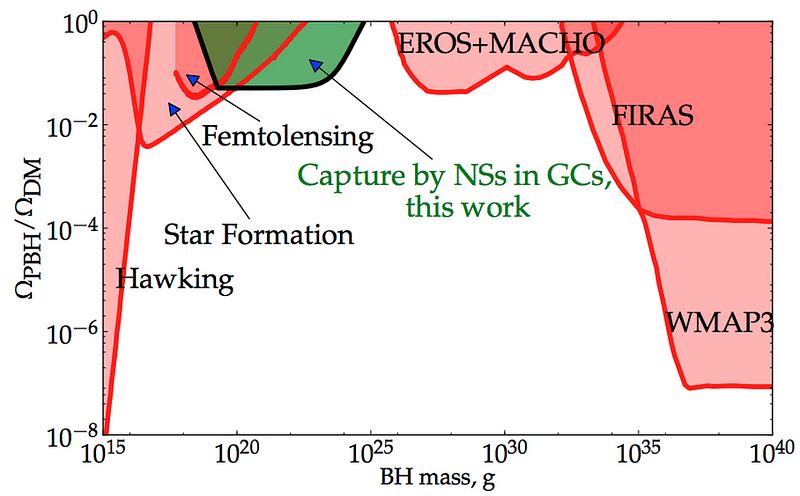
The biggest problem with the Hawking radiation emitted by our Universe’s black holes is the power: the highest-flux black hole emits just 10^-29 W of power, an incredibly small amount. You would have to capture all of the energy emitted via Hawking radiation from the most energetic black hole for four months to equal the energy carried by one typical photon left over, today, from the Big Bang. In terms of signal-to-noise, this is simply not achievable.

The only conceivable way that one could detect Hawking radiation would be to build an enormous, supercooled sphere around the black hole: blocking all the outside radiation and emitting less power from its surface (and, hence, lower-temperature radiation) than the black hole itself emits. It’s a wild idea that’s leaps and bounds beyond any technology imaginable today, although not necessarily impossible. If we ever hope to directly detect Hawking radiation from an actual black hole in our Universe, these are the hurdles we’ll need to overcome.