The Man Who Invented the 26th Dimension
How a scientist you never heard of made String Theory possible.
Image credit: Berkeley Center for Cosmological Physics, at http://aether.lbl.gov/bccp/dimensions.html.
When he died on September 7, 2012, theoretical physicist Claud W. Lovelace left behind a house filled with parakeets. With no family or close companions, the eccentric Rutgers professor loved to be surrounded by his colorful fine-feathered friends and listen to classical music as he contemplated the nuances of unified field theory. A loner not particularly close to his colleagues, members of the Physics and Astronomy department were astounded and delighted when he willed his entire fortune of $1.5 million to it. The funds were used to help establish endowed positions in practical fields of physics, a far cry from his own speculative work. He also willed his collection of more than 4000 classical CDs to Rutgers’ School of the Arts and donated his body to its Medical School.

While Lovelace’s death was little noted in the media—he certainly wasn’t well-known even among physicists outside of string theory—arguably one of his key findings about the high number of dimensions needed for string theory’s consistency had a critical impact on the history of the field. The surprising result established him as one of the most influential theoreticians of the early 1970s. String theorists still grapple with its repercussions.
Let’s step back to 1970, when string theory was in its infancy. While these days we associate strings with attempted “theories of everything,” back then they were used (as the string model) to characterize properties of the strong nuclear force. Today we know that the strong interaction, the force that cements quarks into protons and neutrons, and those, in turn, into atomic nuclei, is conveyed by exchange particles called gluons. Quark-gluon interactions create a situation called confinement that keeps nuclear particles from flying apart: if not for QCD confinement, atomic nuclei would be unstable and we wouldn’t be here.
Before quarks and gluons were identified however, Japanese physicist Yoichiro Nambu and others proposed string theory as a way of explaining the powerful bonds between protons, nucleons, and other particles that experience the strong force, known in general as hadrons. (The theory rendered geometrically an earlier approach by Gabriele Veneziano called dual resonance.) Researchers modeled such bonds as energetic strings that vibrated in different modes, like guitar strings being plucked in various ways and producing different harmonics. That’s when Lovelace entered the scene as a precocious young researcher hoping to make a breakthrough.
Born in England in 1934, Lovelace learned general relativity at the tender age of 16. By then, he and his family had moved to South Africa, where he enrolled at Capetown University. He returned to England in 1958 for his graduate work, conducted at Imperial College, University of London, under the supervision of Pakistani physicist Abdus Salam.

As Lovelace recalled in an email interview I conducted with him in July 2003,
“I was a precocious child. I read Einstein and Dirac at 16-17 and made some very amateurish attempts to construct unified field theories. This probably soured me on them later. Salam, who later shared the Nobel for unifying weak and electromagnetic interactions, was my thesis adviser, but I didn’t take much interest in his wilder speculations.”
Never completing his Ph.D., Lovelace left Imperial for a position at CERN, where he began to explore a vexing issue with hadronic string theory. Researchers had started to use open strings, with loose ends, and closed strings, connected in a loop, to model two types of interactions, known then as Reggeons and Pomerons respectively. To construct a realistic field theory of Pomerons required a property called unitarity: a mathematical condition by which the lengths of vectors are preserved during transformations. A unitary operator spins a vector around an abstract space like a needle twirling on a compass. While the needle turns, it keeps the same length.
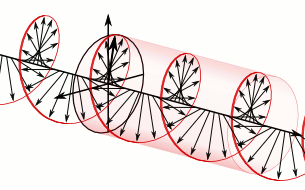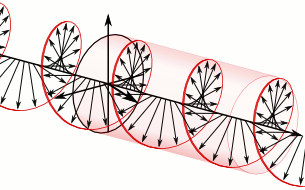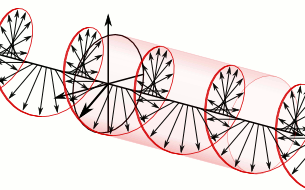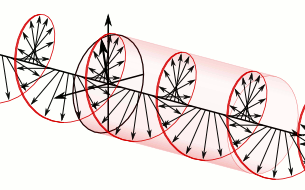
Similarly, unitary operators might change a vector’s components, but its magnitude remains the same. Preserving magnitudes in quantum theory generally means keeping the same overall probabilities and thereby displaying similarly physical properties. Otherwise, strange phenomena might simply appear out of nowhere without physical justification. For that reason, unitarity was a basic requirement of a credible theory.
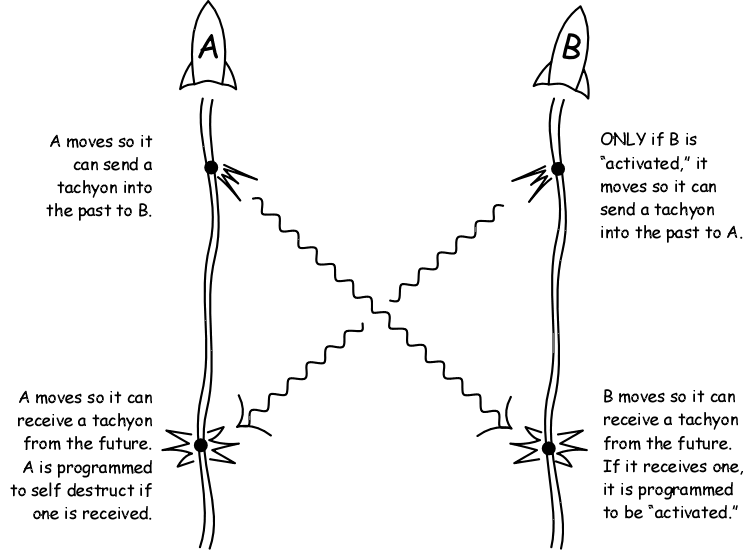
Theorists had tried to no avail to design a Pomeron closed string theory that was unitary in the ordinary four dimensions of spacetime. Instead the theory yielded monstrosities called tachyons that defied the law of cause and effect. A tachyon is a particle or field that travels faster than light and hences moves backward in time. While some researchers such as Gregory Benford have speculated about their properties, they have never been an accepted part of realistic physical theories. Most physicists believe that the only viable way to have a physical theory with tachyons is if they decouple from the theory, meaning they do not impact the observable phenomena — things like cross-sections and scattering amplitudes — that arise from it. (In addition to scholarly papers about tachyons, Benford also wrote a short story called the “Tachyonic Anti-telephone” about causality violations through backwards-in-time communication.)
In a moment of revelation, Lovelace suddenly realized that the solution to the problem was staring him in the face. Suppose one relaxed the assumption that strings lived in a four-dimensional world. He cranked up the dimensions of their surroundings higher and higher, and found that at precisely D = 26 the tachyonic problem vanished and unitarity was restored. He could scarcely believe such an odd result.
He knew that earlier attempts at unifying the laws of nature sometimes made use of an unseen extra dimension. The work of Theodor Kaluza and Oskar Klein independently made use of a fifth dimension in efforts to unite gravitation with electricity in extensions of general relativity. Even Einstein attempted five-dimensional unification during the 1930s and early 1940s, before he abandoned the idea and turned to other unified approaches. However it was quite a leap from 5 dimensions to 26; the latter seemed ridiculously high.
Lovelace gave a talk about his work at a Princeton seminar in December 1970. It did not go well. “I recall it getting a bad reception,” said Lovelace. “I used the 26 dimensions as a joke, and it indeed produced laughter.”
Nevertheless he published his result in a paper entitled “Pomeron form factors and dual Regge cuts.” It appeared in the presigious Physical Review Letters in 1971, guaranteeing a wide audience. Although “thinking it was silly” he had buried the D = 26 result toward the end of the paper, string theorists noticed and were stunned.
“Lovelace’s paper was quite a shock to everyone,” Caltech physicist John Schwarz, who was then at Princeton,” recalled during a talk in 2000, “since until then nobody considered allowing the dimension of spacetime to be anything but four. We were doing hadron physics, after all, and four was certainly the right answer.”

Schwarz became one of the leading developers of superstring theory, a version of string theory that modeled not only forces carriers with strings but also particles. Superstrings utilized supersymmetry—a hypothetical way of transforming fields representing forces into fields representing particles, and the converse. One bonus is that the theory naturally predicted the existence of force carriers with a quantum property called a spin of two. Spin two fields match the features of gravitons, the proposed carriers of the gravitational interaction. For that reason superstrings became seen as a possible avenue toward unifying all of the natural forces: gravity, along with electromagnetism, the strong force and the weak force.
Researchers worked out the dimensionality in which superstring theory would be consistent. It turned out to be D = 10. Perhaps if Lovelace hadn’t produced his earlier result, the thought of looking at such a high number of dimensions would have seemed preposterous. However, with 26 as the comparison, 10 appeared more reasonable. M-theory later added an extra dimension, setting 11 as the norm. All but 4 of these dimensions would be curled up or otherwise inaccessible; that’s why we don’t experience them directly.

Lovelace moved to Rutgers in 1971 and, despite lacking a Ph.D., obtained a professorship. He remained there throughout his entire career, grappling with the nuances of various versions of string theory, while his parakeets played with their own kind of twine. The strain of string quartets piped through his CD player filled his contemplative quarters. While like Einstein, he never achieved unity, he found great joy in the journey.
This post was written by Paul Halpern, Professor of Physics at the University of the Sciences in Philadelphia, PA. Follow Paul’s tweets at @phalpern.
If you enjoyed this, leave your comments on the Starts With A Bang forum here!





