Sorry, Astronomy Fans, The Hubble Constant Isn’t A Constant At All

If your Universe contains any matter at all, a constant Hubble parameter is absolutely impossible.
Our observable Universe is an enormous place, with some two trillion galaxies strewn across the abyss of space for tens of billions of light-years in all directions. Ever since the 1920s, when we first unambiguously demonstrated that those galaxies were well beyond the extent of the Milky Way by accurately measuring the distances to them, one fact leaped out at us: the farther away a galaxy is, on average, the more severely shifted towards the red, long-wavelength part of the spectrum its light will be.
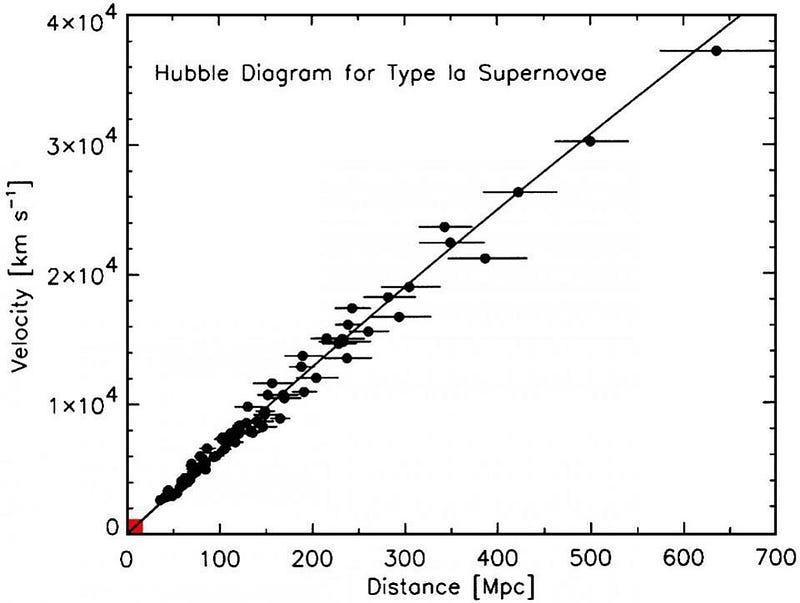
This relationship, between redshift and distance, looks like a straight line when we first plot it out: the farther away you look, the greater the distant object’s redshift is, in direct proportion to one another. If you measure the slope of that line, you get a value, colloquially known as the Hubble constant. But it isn’t actually a constant at all, as it changes over time. Here’s the science behind why.
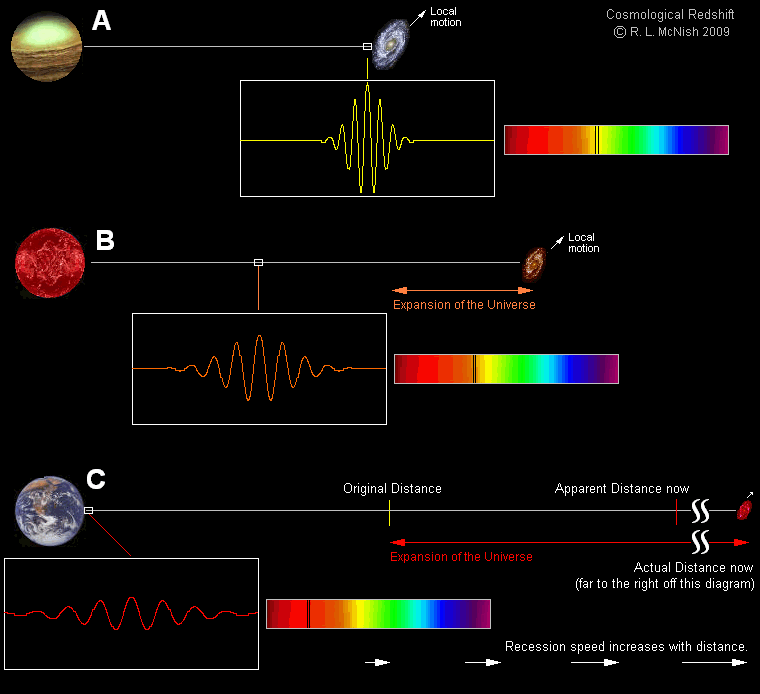
In our Universe, light doesn’t simply propagate through a fixed and unchanging space, arriving at its destination with the same properties it possessed when it was emitted by the source. Instead, it must contend with an additional factor: the expansion of the Universe. This expansion of space, as you can see, above, affects the properties of the light itself. In particular, as the Universe expands, the wavelength of the light passing through that space gets stretched.
If space were expanding at a constant, unchanging rate, then this would account exactly for a constant, unchanging value of “the Hubble constant.” If you, as a photon, traveled through twice the amount of space (or, equivalently, for twice the amount of time) as a closer photon, your wavelength would experience twice the stretch — or redshift — compared to the photon that was closer.
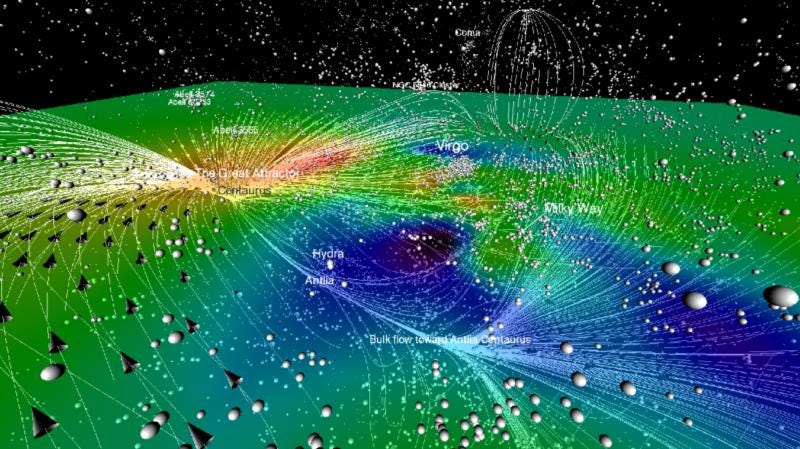
In the real Universe, the relationship isn’t quite as clean as this story, and for good reason: galaxies do more than just stay put in an expanding Universe. Additionally, they experience the gravitational attraction of every other object that’s causally connected to them, pulling them in a variety of different directions at a variety of different speeds.
The notion that the light from a galaxy appears more redshifted the farther away it is from us is only true on average; for any individual galaxy, there will be an additional redshift or blueshift superimposed atop it. That extra signal corresponds to that galaxy’s motion relative to the fabric of space itself, something that astronomers call peculiar velocity. In addition to the effects of the expanding Universe on the light traveling through it, the individual motions of the galaxies themselves — a Doppler shift — affects each individual data point we measure.
But the expansion of space isn’t just an observational phenomeon; it was predicted theoretically before it was ever actually seen. Way back as early as 1922, a Soviet scientist named Alexander Friedmann found a very special solution for the equations governing spacetime in Einstein’s General theory of Relativity.
Friedmann realized that if you assumed that the Universe was, on the largest scales, both isotropic (meaning it was the same no matter which direction you looked in) and homogeneous (meaning it had the same density no matter where you were located), then one can derive two unique equations — the Friedmann equations — that govern the Universe.
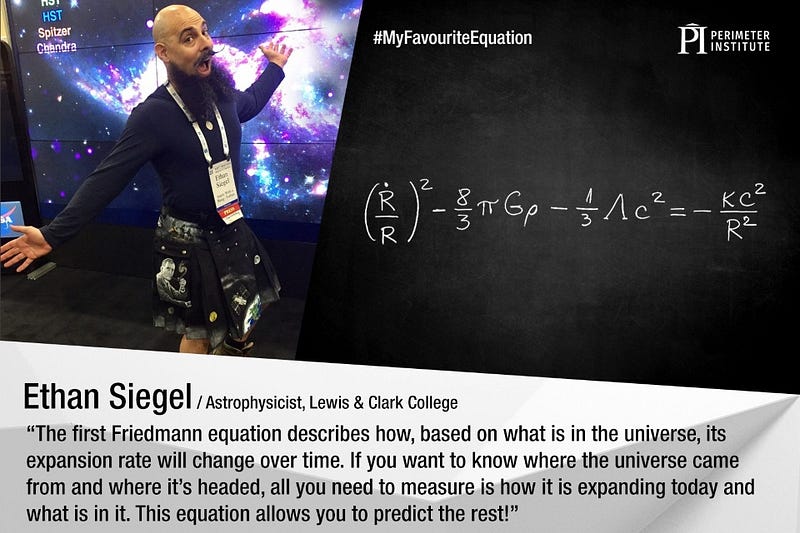
In particular, the most important feature of these equations was that a static Universe is impossible: the Universe must be expanding (or contracting), and therefore, the light from distant objects must be redshifted (or blueshifted) accordingly. These equations were later derived by multiple scientists independently: Georges Lemaître, Howard Robertson, and Arthur Walker all have their names attached to various underlying components of how these equations were obtained.
But the biggest feature you should notice about this equation is simple: there are two sides to it, the left-hand side and the right-hand side. On the left is the expansion rate of the Universe — what we’ve been calling the Hubble constant — and on the right is a series of terms that correspond to the various densities of all the forms of matter and energy present within that same Universe.
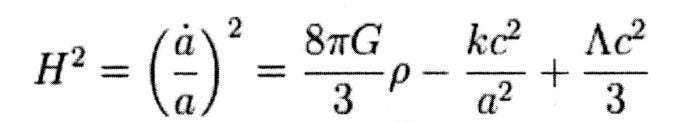
Now, here’s the important thing you have to think about: when the Universe expands, what happens to a quantity like matter density or energy density? The correct answer is, “it depends on what type of matter or energy you have.” For example, as the Universe expands, its volume increases, but the total number of particles within it remains the same. Radiation, like photons, also gets stretched to longer wavelengths (and lower energies), while dark energy, which is a form of energy inherent to the fabric of space itself, has a constant energy density even as the Universe expands.
As time goes on, the volume of an expanding Universe increases, which means, at a base level, that the energy densities of all the individual components combined are not required to remain constant. In fact, in almost all cases, they will not.
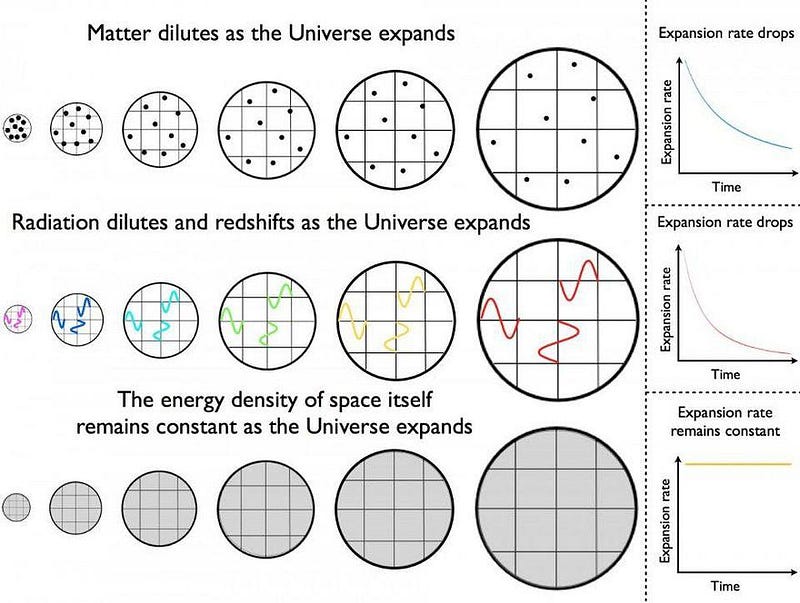
Because of what the Friedmann equations tell us, we know that a Universe with a greater energy density will expand at a faster rate, while one with a smaller energy density must expand at a slower rate. So long as the energy density does not remain the same at all times, the expansion rate must change as well. The big question, of how the expansion rate evolves with time, is entirely dependent on what exists within our Universe.
There are many possible ingredients that can exist in an expanding Universe, and each one will evolve according to the unique properties inherent to that particular form of energy. Radiation and neutrinos were the most important ingredients, energy-wise, a very long time ago, later replaced by normal matter and dark matter as the dominant ingredients. As we move far into the future, dark energy will dominate, eventually causing the Hubble rate to asymptote to a finite, non-zero value.
In fact, the most useful part of the relationship between the expansion rate and the Universe’s contents is that it gives us a method to go out and physically measure two things simultaneously:
- how quickly the Universe is expanding at the present,
- and what the relative values of the different significant components of the energy density are, both today and in the past.
Think about it this way: the light that arrives at our eyes, today, had to travel through the expanding Universe to get there. Light that arrives from a nearby galaxy was only emitted a short time ago, and the Universe’s expansion rate has only changed by a small amount in that time. Therefore, the nearby Universe gives us a handle on the present expansion rate. However, light that requires a journey of many billions of years to reach us will see the expansion rate change over time.
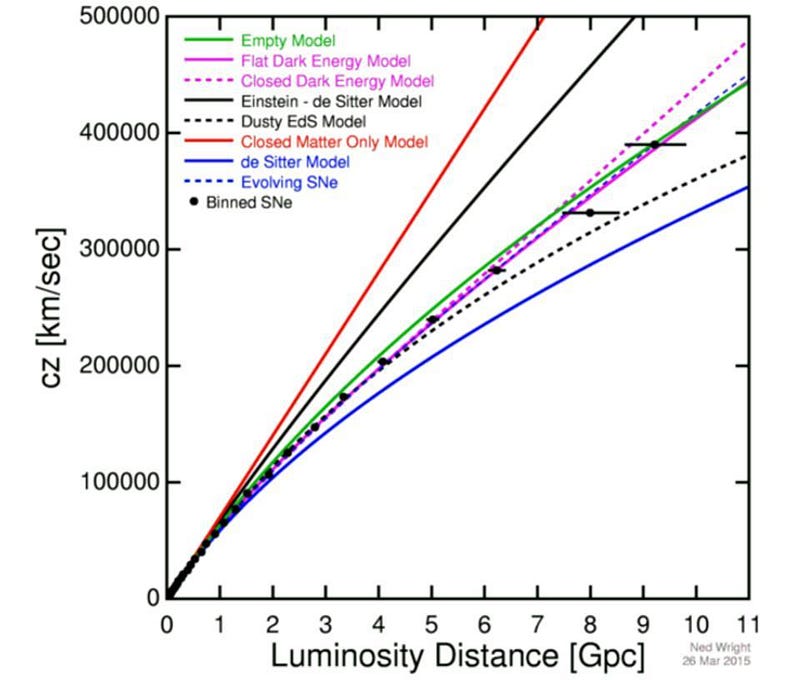
By making measurements of galaxies at a wide variety of distances, we can determine what the expansion rate was (and how it changed) over many billions of years. Those changes in the expansion rate of the Universe teach us what the different components that make up the Universe are, as all the light traveling through the Universe will experience the expansion of space.
This also motivates us to measure light from progressively farther, more distant objects. If we want to understand how the Universe came to be the way it is today, and how the expansion rate has evolved, the best thing we can do it to measure how light redshifts as it travels to us throughout our entire cosmic history. With everything we’ve measured today, we can not only reconstruct what our Universe is made of now, but what it was made of at every point throughout our past.
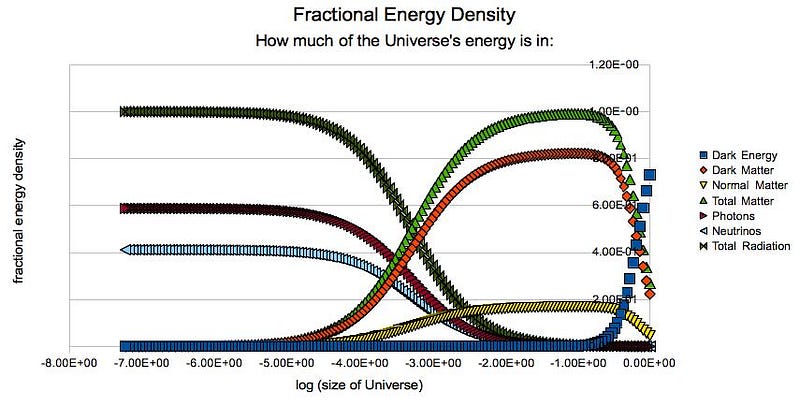
The fact that the Hubble expansion rate of the Universe changes over time teaches us that the expanding Universe isn’t a constant phenomenon. In fact, by measuring how that rate changes over time, we can learn what our Universe is made from: this was exactly how dark energy was first discovered.
But the “Hubble constant” itself is a misnomer. It has a value today that’s the same everywhere in the Universe, making it a constant in space, but it’s not a constant in time. In fact, so long as matter remains in our Universe, it will never become a constant, as increasing the volume will always make the density (and, a la Friedmann, the expansion rate) decrease. Perhaps it’s time to call it by its more accurate but rarely used name: the Hubble parameter. Its present value is not a constant either, and should perhaps be called the Hubble parameter today. As it changes with time, it continues to reveal the very nature of our expanding Universe.
Ethan Siegel is the author of Beyond the Galaxy and Treknology. You can pre-order his third book, currently in development: the Encyclopaedia Cosmologica.





