Some Black Holes Are Impossible In Our Universe

Enough mass in one location will always create a black hole. But not all masses are possible.
If you take enough mass and compress it into a small enough volume of space, you’ll inevitably form a black hole. Any mass in the Universe will curve the fabric of spacetime around it, and the more severely curved that spacetime fabric is, the more difficult it is to escape from that mass’s gravitational pull. The smaller the volume becomes that your mass occupies, the faster you’d have to travel, at the edge of that object, to actually escape it.
At some point, the escape velocity you’d need to obtain would exceed the speed of light, which defines the critical threshold for forming a black hole. According to Einstein’s General Relativity, any mass in a small enough volume would be sufficient to form a black hole. But in our physical reality, there are real limitations that our Universe is subjected to, and not every mathematical possibility comes to fruition. Many of the black holes that we could imagine forming simply don’t in our Universe. To the best of our knowledge, here’s what’s impossible.
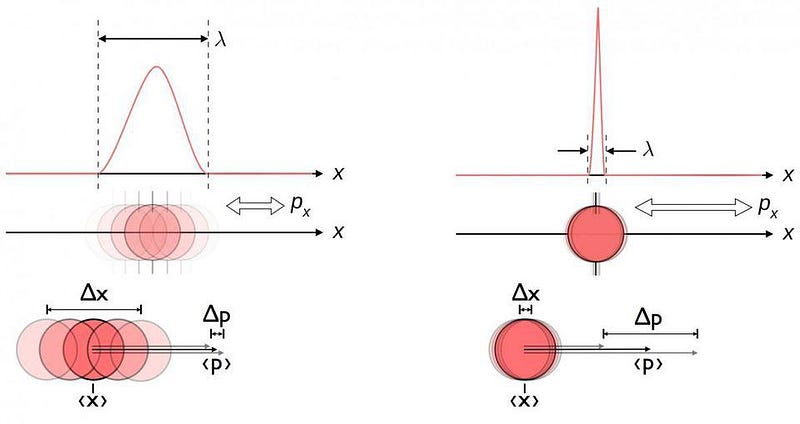
Black holes have a quantum limit. Below a certain scale, reality is not what it seems. Instead of matter and energy having specific properties that are limited only by our ability to measure it, we’ve found that there are inherently uncertain relationships between various properties. If you measure a particle’s position, you’ll know its uncertainty inherently less well. If you measure its lifetime or its behavior over extremely short timescales, the less well-known you can inherently know its intrinsic energy, or even its rest mass.
There’s an inherent limit to how well you can know any two complementary quantities simultaneously, which is the key point of the Heisenberg uncertainty principle. Even empty space — if you were to remove all the various forms of matter and energy entirely — exhibits this uncertainty. Well, if you consider a distance scale of ~10^-35 m or smaller, the amount of time it would take a photon to cross it would be minuscule: ~10^-43 s. On those short timescales, the Heisenberg uncertainty principle tells you that your energy uncertainty is so large, it corresponds (via E = mc²) to a mass of about 22 micrograms: the Planck mass.

If you had a black hole — a perfect singularity — whose mass was 22 micrograms, how large would its event horizon be? The answer is that same distance scale (the Planck length) you started off with: ~10^-35 m. This fact illustrates why physicists say that the laws of reality “break down” at the Planck scale: the quantum fluctuations that must spontaneously occur are so large in magnitude, on scales so minuscule, that they’re indistinguishable from black holes.
But those black holes would immediately decay, as the evaporation time due to Hawking radiation would be less than the Planck time: ~10^-43 s. We know that the laws of physics we have, both in quantum physics and in General Relativity, cannot be trusted on these small distance scales or on these tiny timescales. If that’s true, then we cannot accurately describe, with those same equations, a black hole whose mass is 22 micrograms or lower. That’s the quantum lower limit for how small a black hole can be in our Universe. Below it, any assertion we could make would be physically meaningless.

Black holes below a certain mass would all have evaporated away by now. One of the remarkable lessons from applying quantum field theory in the space around black holes is this: black holes aren’t stable, but will emit energetic radiation, eventually leading to their complete evaporation. This process, known as Hawking radiation, will someday cause every black hole within the Universe to evaporate.
Although there’s a lot of confusion around why this happens — much of which can be traced back to Hawking himself — the key things you must understand are that:
- the radiation is caused by the difference in spacetime’s curvature near and far away from the black hole’s event horizon,
- and that the lower in mass your black hole is, the smaller its event horizon is, and therefore the larger the spatial curvature is at that critical location in space.
As a result, lower-mass black holes evaporate more quickly than higher-mass ones. If our Sun were a black hole, it would take 10⁶⁷ years to evaporate; if the Earth were one, it would evaporate much more quickly: in just ~10⁵¹ years. Our Universe, since the hot Big Bang, has existed for about 13.8 billion years, meaning any black holes less massive than ~10¹² kg, or around the mass of all the humans on Earth combined, would already have evaporated away entirely.
Black holes below about ~2.5 solar masses probably don’t exist. According to the laws of physics as we understand them, there are only a few ways that a black hole can be formed. You can take a large chunk of matter and let it gravitationally collapse; if there’s nothing to stop or slow it down, it could collapse directly into a black hole. You could, alternatively, let a clump of matter contract down to form a star, and if that star’s core is massive enough, it can eventually implode, collapsing down to form a black hole. Finally, you can take a stellar remnant that didn’t quite make it — like a neutron star — and add mass, either through a merger or accretion, until it becomes a black hole after all.
In practice, we believe all of these methods occur, leading to the formation of the realistic black holes that form in our Universe. But below a certain mass threshold, none of these methods can actually give you a black hole.
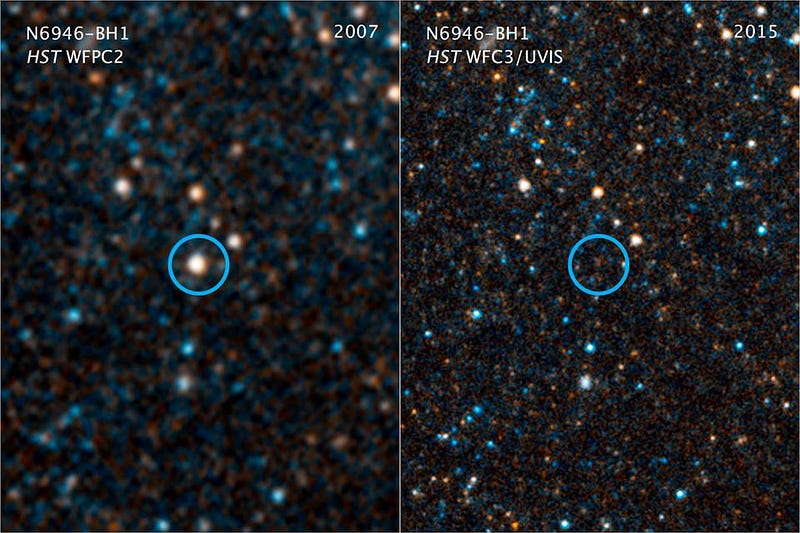
We’ve seen clumps of matter suddenly “wink out” of existence, like stars that magically disappear. The most logical explanation, as well as the one that best fits the data, is that a fraction of stars do spontaneously collapse into a black hole. Unfortunately, they tend to be on the massive side: dozens of times as massive as our Sun at the very least.
Stars with massive cores do often end their lives in spectacular supernova explosions, where the cores of these stars do implode. If you’re born with about 800% or more of our Sun’s mass, you’re an excellent candidate for going supernova. The stars with less massive cores will eventually form neutron stars, with the more massive ones forming black holes. The heaviest neutron star ever discovered likely formed through this process, weighing in at 2.17 solar masses.
And finally, you can take object that are lighter than black holes — like the aforementioned neutron stars — and either allow them to accrete/siphon mass from a companion, or collide them with another massive, compact object. When they do, there’s a chance they could form a black hole.
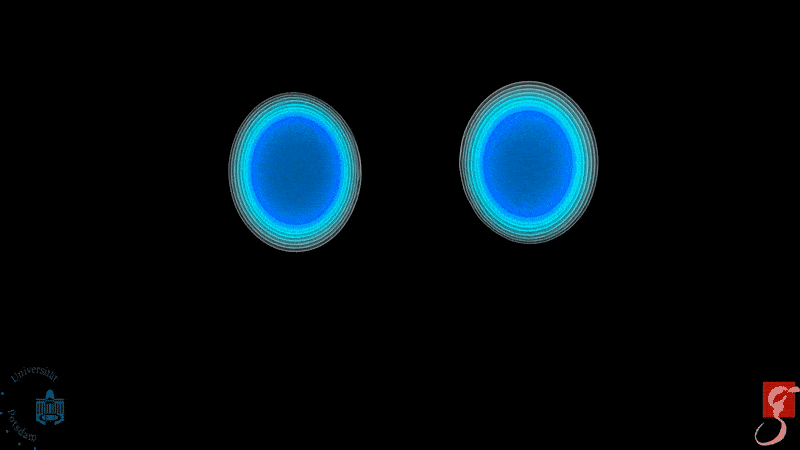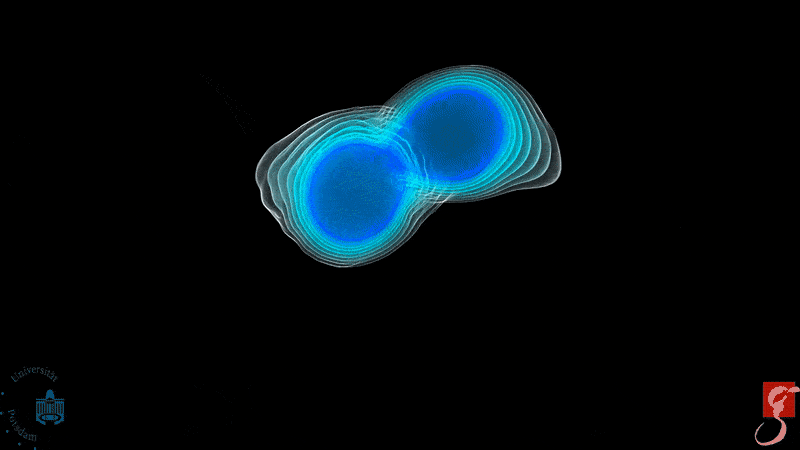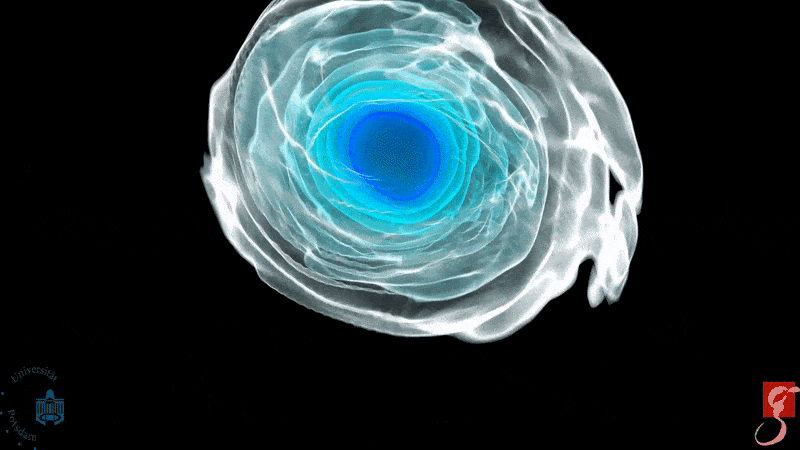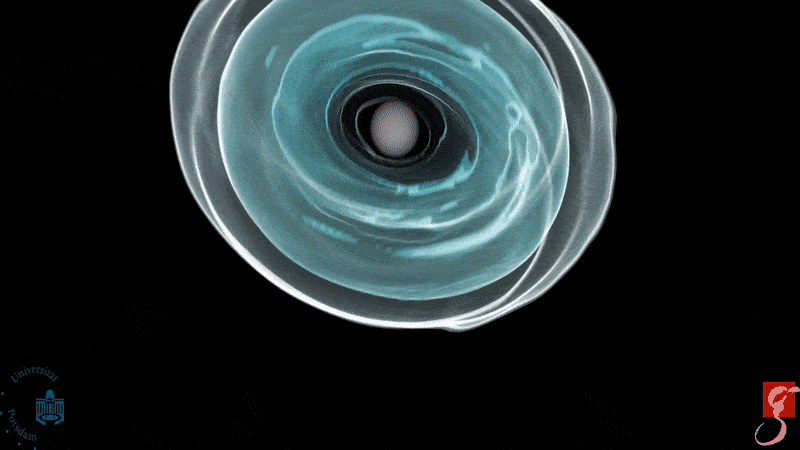
Although there have been only two neutron star-neutron star mergers ever directly and definitively observed, they’ve been incredibly informative. The second one, with a combined mass of about 3.4 solar masses, went directly to a black hole. But the first one, which had a combined mass of more like 2.7 solar masses, revealed a far more complex story. For a few hundred milliseconds, this rapidly-spinning, post-merger mass behaved like a neutron star. All of a sudden, however, it switched to behaving like a black hole. After that transition, it never went back.
What we now believe occurred is that there’s a narrow mass range — somewhere between 2.5 and maybe 2.8 solar masses — where a collapsed objects like a neutron star can exist, but it requires a particularly high value for its rotation rate. If it drops below a critical value, and it will change its spin rate as it settles down to a more spherical shape, it will become a black hole. Below that lower value, there are only neutron stars and no black holes. Above that upper value, there are only black holes and no neutron stars. And in between, you can have both, but what you’ll ultimately wind up with depends on how fast the object is spinning.
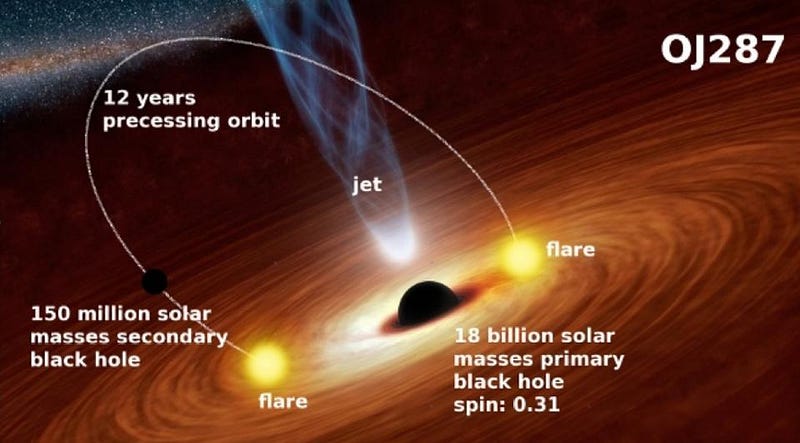
What about heavier black holes? Is there a ‘gap’ where no black holes exist? Is there an upper limit to black hole masses? Black holes can get much, much heavier than just a few times the mass of our Sun. Initially, there were theoretical concerns that there might be a “gap” where black holes didn’t exist; that appears to conflict with the data we now have after ~6 years of advanced LIGO. There was a worry that intermediate mass black holes might not exist, as they’ve proven very difficult to find. However, they now appear to be out there as well, with superior data confidently revealing numerous examples.
There will be a limit to how big they can get, however, although we haven’t hit it just yet. Black holes approaching 100 billion solar masses have been found, and we even have our first candidate for crossing that vaunted threshold. As galaxies evolve, merge, and grow, so too can their central black holes. Far into the future, some galaxies may grow their black holes as large as ~100 trillion (10¹⁴) solar masses: 1000 times larger than today’s largest black hole. Owing to dark energy, which drives distant galaxies apart in the expanding Universe, we fully expect that no black holes will ever grow substantially larger than this value.
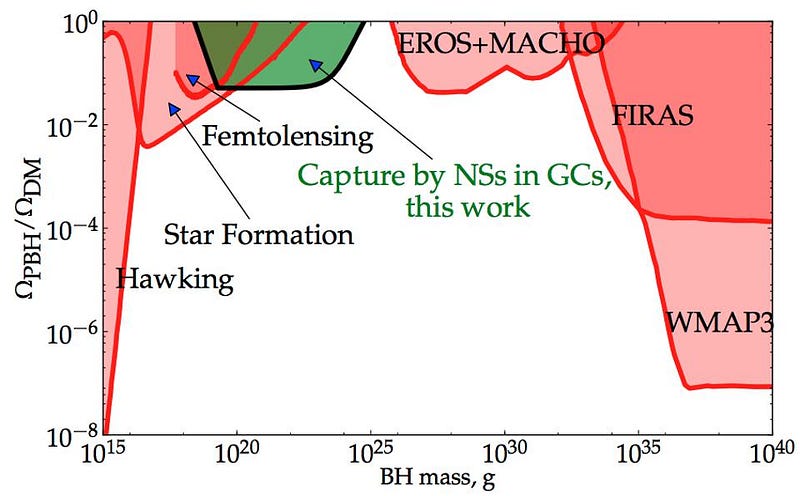
What about primordial black holes: black holes that formed directly after the Big Bang? This is a sticky one, because there’s no evidence that they exist. Observationally, many constraints have been placed on the idea, which has been around since the 1970s. When the Universe was born, we know some regions were denser than others. If one region was born with a density that was just ~68% greater than average, that entire region should inevitably collapse to form a black hole. While their masses can’t be less than ~10¹² kg, they could, in theory, have any value that’s larger.
Unfortunately, we have the fluctuations in the cosmic microwave background to guide us. These temperature fluctuations correspond to the overdense and underdense regions in the early Universe, and show us that the overdense regions are only about ~0.003% denser than average. It’s true: these are on larger scales than the ones we’d look for black holes on. But with no compelling theoretical motivation for them, and no observational evidence in their favor, this idea remains purely speculative.
For a long time, the very notion of black holes was highly contentious. For about 50 years after they were first derived in General Relativity, no one was sure whether they could physically exist in our Universe. Roger Penrose’s Nobel-winning work demonstrated how their existence was possible; just a few years later, we discovered the first black hole in our own galaxy: Cygnus X-1. Now the floodgates are open, with stellar-mass, intermediate-mass, and supermassive black holes all known in great and ever-increasing numbers.
But there’s a lower limit to black holes in the Universe: we believe that none exist below about 2.5 times the mass of the Sun. Additionally, while the heaviest black holes today are right around 100 billion solar masses, they’ll eventually grow to be up to 1000 times as heavy as that. Studying black holes provides us with a unique window into the physics of our Universe and the nature of gravity and spacetime themselves, but they can’t reveal everything. In our Universe, some black holes truly are impossible.
Starts With A Bang is written by Ethan Siegel, Ph.D., author of Beyond The Galaxy, and Treknology: The Science of Star Trek from Tricorders to Warp Drive.





