Is The Matter In Our Universe Fundamentally Stable Or Unstable?

If we waited long enough, would even protons themselves decay?

There are certain things in the Universe that, if you leave them alone for long enough, they’ll eventually decay away. Other things, no matter how long we wait, have never been observed to decay. This doesn’t necessarily mean that they’re stable, only that if they’re unstable, they live longer than a certain measurable limit. While a large number of the particles — both fundamental and composite — are known to be unstable, there are a select few that appear to be stable, at least so far, to the precision we’ve been able to measure.

But are they truly, perfectly stable, destined never to decay even as the cosmic clock runs forward for all eternity? Or, if we could wait long enough, would we eventually see some or even all of those particles eventually decay away? And what does it mean for the Universe if a previously-thought-to-be-stable atomic nucleus, an individual proton, or even fundamental particles like the electron, a neutrino, or the photon turn out to decay? Here’s what it would mean if we lived in a Universe where our matter was fundamentally unstable.

The internal structure of a proton, with quarks, gluons, and quark spin shown. The nuclear force acts like a spring, with negligible force when unstretched but large, attractive forces when stretched to large distances. To the best of our understanding, the proton is a truly stable particle, and has never been observed to decay. (BROOKHAVEN NATIONAL LABORATORY)

It’s actually a relatively novel idea that any form of matter would be unstable: something that only arose as a necessary explanation for radioactivity, discovered in the late 1800s. Materials that contained certain elements — radium, radon, uranium, etc. — appeared to spontaneously generate their own energy, as though they were powered by some sort of internal engine inherent to their very nature.

Over time, the truth about these reactions was uncovered: the nuclei of these atoms were undergoing a series of radioactive decays. The three most common types were:

α (alpha) decay: where an atomic nucleus spits out an α-particle (with 2 protons and 2 neutrons), moving down 2 elements on the periodic table,
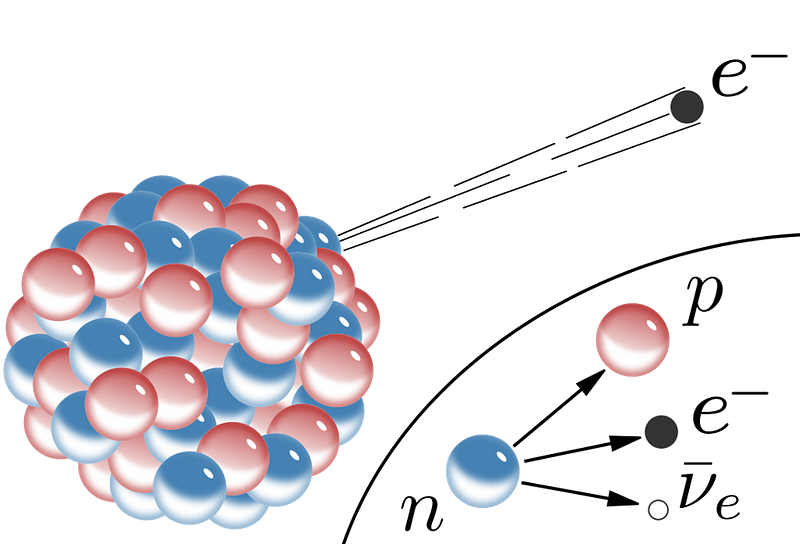
β (beta) decay: where an atomic nucleus converts a neutron into a proton while spitting out an electron (a β-particle) and an anti-electron neutrino, moving up 1 element on the periodic table,
γ (gamma) decay: where an atomic nucleus, in an excited state, spits out a photon (a γ-particle), transitioning to a lower-energy state.

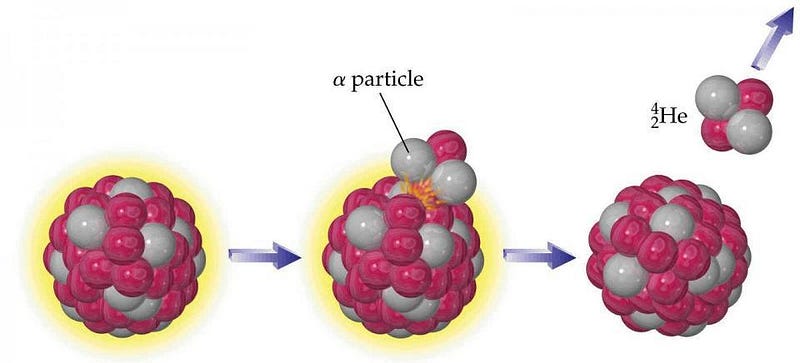
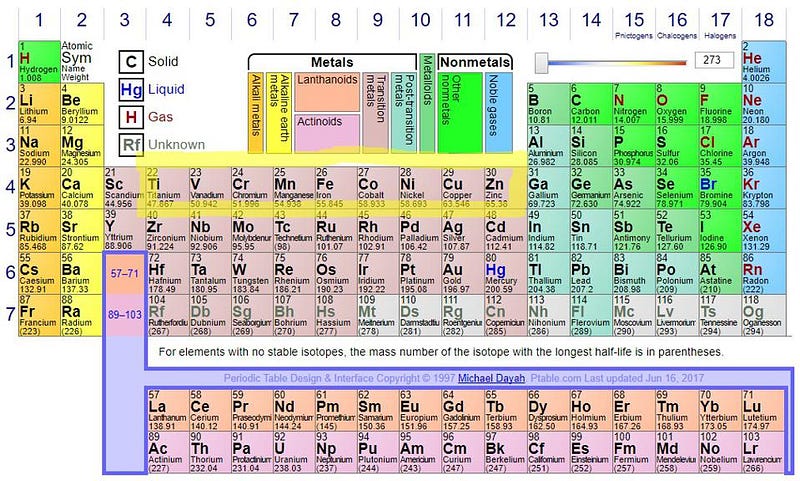
An alpha-decay is a process where a heavier atomic nucleus emits an alpha particle (helium nucleus), resulting in a more stable configuration and releasing energy. Alpha decay, along with beta and gamma decays, are the main ways by which naturally occurring elements undergo radioactive decay. (NUCLEAR PHYSICS LABORATORY, UNIVERSITY OF CYPRUS)

At the end of these reactions, the total mass of what’s left over (the products) is always less than the total mass of what we began with (the reactants), with the remaining mass converted into pure energy via Einstein’s famous equation, E = mc². If you learned about the periodic table prior to 2003, you probably learned that bismuth, the 83rd element, was the heaviest stable element, with every element heavier than that undergoing some form of radioactive decay (or decay chain) until a truly stable element is reached.

But in 2003, scientists discovered that every single isotope of bismuth is inherently unstable, including the abundant, naturally occurring bismuth-209. It’s extremely long-lived, with a half-life of around ~10¹⁹ years: approximately one billion times the age of the present Universe. Since that discovery, we now report that lead, the 82nd element, is the heaviest stable element. But given enough time, it’s possible that it will decay, too.

Although Bismuth is still considered to be ‘stable’ by many, it is fundamentally unstable and will undergo alpha decay on timescales of around ~1⁰¹⁹ years. Based on experiments conducted in 2002 and published in 2003, the periodic table has been revised to indicate that lead, not bismuth, is the heaviest stable element. (MICHAEL DAYAH / HTTPS://PTABLE.COM/)

The reason that radioactive decays occur wasn’t well understood for many decades after the discovery of radioactivity: it’s an inherently quantum process. There are certain conservation rules that are an inextricable part of the laws of physics, as quantities like energy, electric charge, and linear and angular momentum are always conserved. That means, if we were to measure those properties for both the reactants and the products (or the physically possible products) of any candidate reaction, they must always be equal. These quantities cannot be spontaneously created or destroyed; that’s what it means to be “conserved” in physics.

But if there are multiple configurations that are allowed that obey all of those conservation rules, some of them will be more energetically favorable than others. “Energetically favorable” is like being a round ball on top of a hill and rolling down it. Where will it come to rest? At the bottom, right? Not necessarily. There can be many different low-points where the ball can wind up, and only one of them will be the lowest.

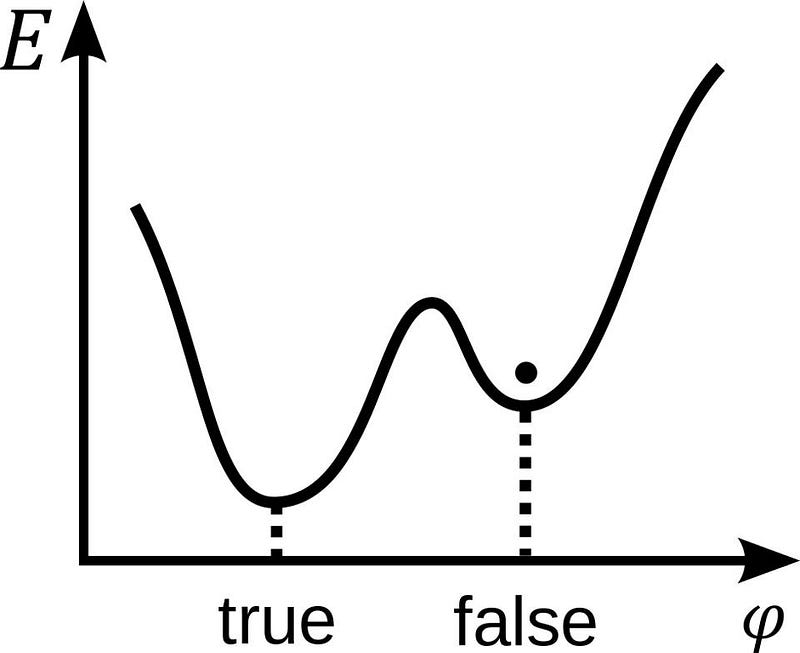
A scalar field φ in a false vacuum. Note that if you roll down a hill, you can wind up in the “false” vacuum instead of the true vacuum. Classically, you’d have to give a particle in the false vacuum state enough energy to jump up over that barrier, but in the quantum universe, it’s possible to tunnel directly into the true vacuum state. (WIKIMEDIA COMMONS USER STANNERED)

In classical physics, if you get trapped in one of these “false minima,” or a low-point that isn’t the lowest possible configuration, you’ll be stuck there unless something comes along to give that ball enough energy to rise above the boundaries of the pit it’s in. Only then will it have the opportunity to begin its descent down the hill anew, with the potential to eventually make it to a lower-energy configuration, possibly winding up in the lowest-energy (ground) state of all.

But in quantum physics, you don’t need to add energy for that transition to become possible. Instead, in the quantum Universe, it’s possible to spontaneously jump from one of those false minimum states to a lower-energy configuration — even directly into the ground state — without any external energy at all. This phenomenon, known as quantum tunneling, is a probabilistic process. If the laws of nature don’t explicitly forbid such a process from occurring, then it most definitely will. The only question is how long it will take.

The transition across a quantum barrier is known as quantum tunneling, and the probability of a tunneling event occurring in a given amount of time is dependent on a variety of parameters about the energies of the products and reactants, the interactions that are allowed between the particles involved, and the number of allowable steps required to arrive at the end state. (AASF / GRIFFITH UNIVERSITY / CENTRE FOR QUANTUM DYNAMICS)

In general, there are a few main factors that determine how long an unstable (or quasi-stable) state will last.

What is the energy difference between the reactants and the products? (Bigger differences, and bigger percentage differences, translate to shorter lifetimes.)
How suppressed is the transition from your current state to the final state? (I.e., what’s the magnitude of the energy barrier?)
How many “steps” does it take to get from the initial state to the final state? (Fewer steps lead to a more likely transition.)
And what is the nature of the quantum pathway that gets you there?

A particle like a free neutron is unstable, as it can undergo β decay, transitioning to a proton, an electron, and an anti-electron neutrino. (Technically, one of the down quarks inside β-decays into an up quark.) A different quantum particle, the muon, is also unstable and also undergoes β-decay, transitioning to an electron, an anti-electron neutrino, and a muon neutrino. They’re both weak decays, and both mediated by the same gauge boson. But because the products of neutron decay are 99.9% the mass of the reactants, while the products of muon decay are only ~0.05% of the reactants, the muon’s mean lifetime is measured in microseconds, while a free neutron lives for about ~15 minutes.

Schematic illustration of nuclear beta decay in a massive atomic nucleus. Beta decay is a decay that proceeds through the weak interactions, converting a neutron into a proton, electron, and an anti-electron neutrino. The free neutron lives for about ~15 minutes as a mean lifetime, but bound neutrons can be stable for as far as we’ve ever measured them. (WIKIMEDIA COMMONS USER INDUCTIVELOAD)

Measuring unstable particles individually is an excellent method for determining their properties so long as they’re short-lived compared to human timescales. You can observe them one-at-a-time and see how long they last until they eventually decay away. But for particles that live for extremely long times — longer even than the age of the Universe — that approach won’t work. If you took a particle like bismuth-209, and waited for the entire age of the Universe (~10¹⁰ years), there’s less than a 1-in-a-billion chance that it would decay. It’s a terrible approach.

But if you took an enormous number of bismuth-209 particles, like Avogadro’s number of them (6.02 × 10²³), then after a year a little more than 30,000 of them would decay. If your experiment was sensitive enough to measure that tiny change in the atomic composition of your sample, you’d be able to detect and quantify just how unstable bismuth-209 is. This idea was a critical test for an important idea in particle physics back in the 1980s: grand unified theories.

An equally-symmetric collection of matter and antimatter (of X and Y, and anti-X and anti-Y) bosons could, with the right GUT properties, give rise to the matter/antimatter asymmetry we find in our Universe today. In grand unified theories, additional new particles that couple to Standard Model particles, such as the X and Y bosons shown here, would inevitably lead to proton decay, which must be suppressed to agree with observations. (E. SIEGEL / BEYOND THE GALAXY)

In our current, low-energy Universe, we have four fundamental forces: the gravitational force, the electromagnetic force, and the strong and weak nuclear forces. At high energies, two of those forces — the electromagnetic force and the weak nuclear force — unify and become a single force: the electroweak force. At still higher energies, based on important ideas from group theory in particle physics, it’s theorized that the strong nuclear force unifies with the electroweak force. This idea, called grand unification, would have important consequences for a vital building block of matter: the proton.

Under the Standard Model alone, there’s no good pathway for the proton to decay; its lifetime should be so long that if we monitored every proton in the Universe for the lifetime of the Universe since the Big Bang, exactly zero of them should decay. But if grand unification is correct, then the proton should easily be able to decay into pions and (anti-)leptons, and should have a lifetime of “merely” ~10³⁰ years in the simplest model. That might seem unfathomably long, but physicists have a way to test this.

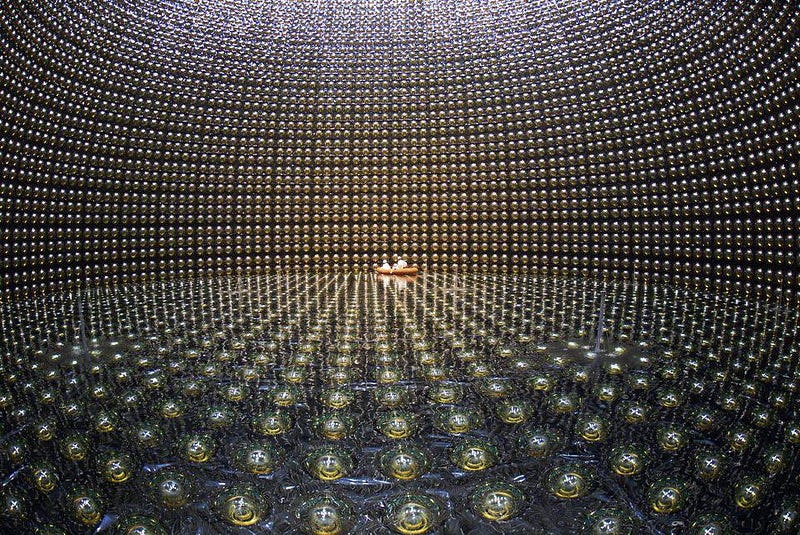
Experiments such as Super-Kamiokande, which contain enormous tanks of (proton-rich) water surrounded by arrays of detectors, are the most sensitive tool humanity has to search for proton decay. As of the start of 2020, we only have constraints on potential proton decay, but there is always the potential for a signal to emerge at any time. (KAMIOKA OBSERVATORY, ICRR (INSTITUTE FOR COSMIC RAY RESEARCH), THE UNIVERSITY OF TOKYO)

All you have to do is gather enough protons — such as from the hydrogen atoms in a water molecule — together in one place, and build a sensitive enough suite of detectors to identify the telltale signal that would emerge if protons decayed. If you get 10³⁰ of them together and wait for a year, you should be able to measure their half-life if it’s shorter than 10³⁰ years, and place a lower limit on their lifetime otherwise. After decades of these experiments, combined with the information we learn about proton lifetimes from neutrino detector experiments, we now know the proton’s lifetime can be no shorter than around ~10³⁵ years.

That tells us that the simplest grand unified theories cannot reflect our reality, but it doesn’t tell us whether the proton is truly stable or not. Similarly, “stable” atomic nuclei may someday decay; electrons, neutrinos, and photons may someday decay; even gravitational waves or space itself may not be eternal. Some of our strongest constraints on beyond-the-Standard-Model physics come from the non-observation of these and other decays. To the limits of what we’ve measured, most of the Universe’s components appear stable.

Because bound states in the Universe are not the same as completely free particles, it may be conceivable that the proton is less stable than we observe it to be by measuring the decay properties of atoms and molecules, where protons are bound to electrons and other composite structures. With all the protons we’ve ever observed in all our experimental apparatuses, however, we’ve never once seen an event consistent with proton decay. (GETTY IMAGES)

But is the matter in our Universe truly stable in some form, or will it all eventually — if we wait for arbitrarily long times — decay in some way? It’s important to remember that what we’re measuring with our experiments is limited to how we’re performing our experiments.

Travel the Universe with astrophysicist Ethan Siegel. Subscribers will get the newsletter every Saturday. All aboard!

For example, a free neutron has a mean lifetime of ~15 minutes, but a neutron in a neutron star has enough binding energy that it’s entirely stable: it can never decay. Similarly, it’s possible that protons or certain atomic nuclei really are intrinsically unstable, but because we’re measuring them as they are bound in atoms and molecules, we see them as stable. Our conclusions are only as good as the experiments used to reach them.

Two possible pathways for proton decay are spelled out in terms of the transformations of its fundamental constituent particles. These processes have never been observed, but are theoretically permitted in many extensions of the Standard Model, such as SU(5) Grand Unification Theories. (JORGE LOPEZ, REPORTS ON PROGRESS IN PHYSICS 59(7), 1996)

Nevertheless, the fact that we’ve measured the stability of so many fundamental and composite particles gives us the strongest constraints of all, in many ways, on possible modifications to the Standard Model. Simple models of grand unification are ruled out. Many supersymmetric theories are completely dead. Other ideas that introduce new particles, including technicolor theories and theories involving extra dimensions, are restricted by the observed stability of the matter in our Universe.

Even though the ultimate fate of the matter in our Universe has yet to be determined, the wiggle room is already narrower than many of the greatest ideas that 20th and 21st century physicists have been able to concoct. We may not know everything about what the Universe is, but it’s impressive how much we know about what the Universe isn’t.

Starts With A Bang is written by Ethan Siegel, Ph.D., author of Beyond The Galaxy, and Treknology: The Science of Star Trek from Tricorders to Warp Drive.