Did Han Solo Use A Trick Of Einstein’s Relativity To Make The Kessel Run?

As the new Star Wars movie, Solo, premieres, let’s look at the physics of how his most famous achievement might actually be possible.

The Millennium Falcon’s greatest achievement, according to its captain in Star Wars: A New Hope, seemed to defy the laws of physics itself. When Han Solo, the ship’s captain, met Luke Skywalker and Obi-Wan Kenobi, he seemed incredulous that the two men wouldn’t know that the ship is fast enough to outrun the Empire. “You’ve never heard of the Millennium Falcon,” Solo questions. “It’s the ship that made the Kessel Run in less than twelve parsecs.” Obi-Wan looks incredulously at Solo, perhaps unmoved by the illogical boast.

It’s illogical, of course, because when you boast about a vehicle’s speed, you typically speak about how quickly it can get you to your destination. You might be impressed to make something called the “Kessel Run” in less than 30 minutes, but you wouldn’t be impressed if you made it in less than 30 miles. That’s what a parsec is: a unit of distance, approximately equal to 3.26 light years.

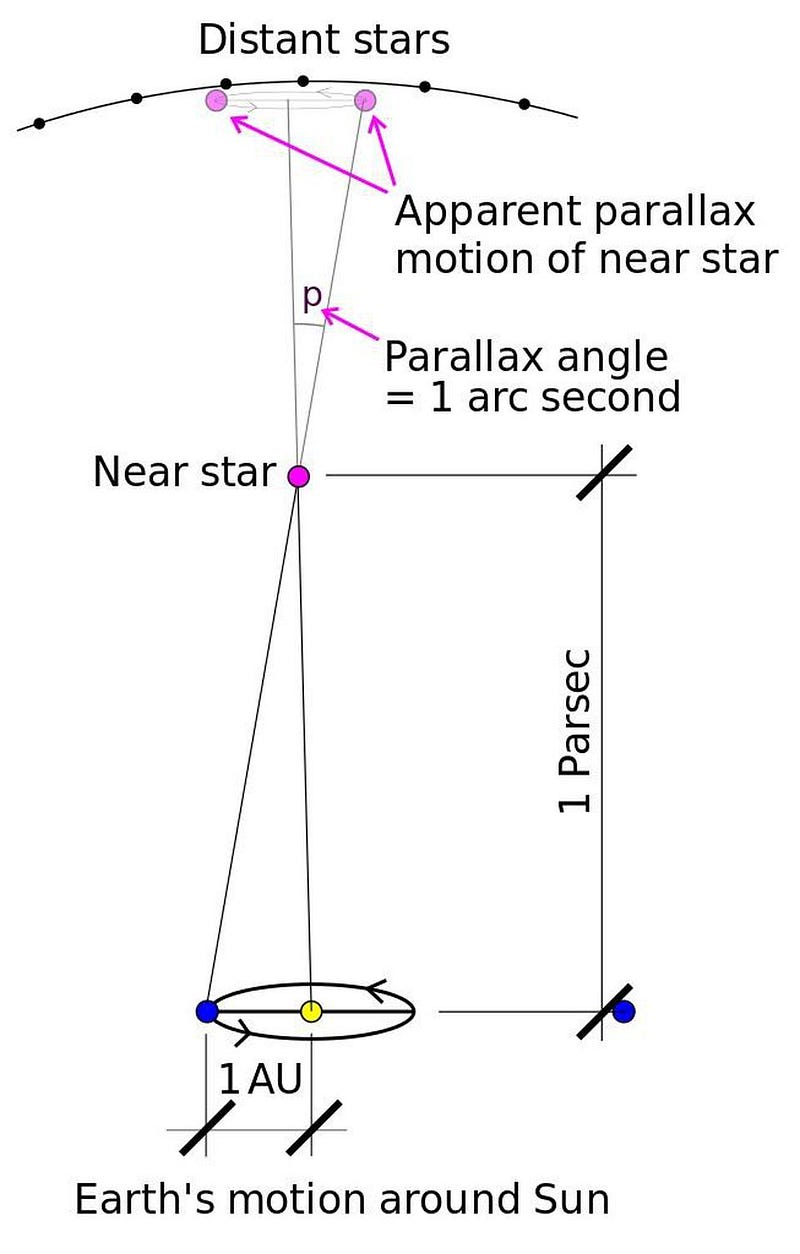
But perhaps there’s some good logic there after all. Typically, the shortest distance between two points is a straight line, and perhaps the standard path you’d have to travel to make the Kessel Run could be something closer to 18 parsecs. But in Einstein’s General Relativity, there can be even shorter distances between two points than a straight line; sometimes a particular curved path is superior. Especially when space is highly curved, such as in the presence of very massive objects, a short-cut through space may well be possible.

According to modern astrophysics, here’s how the Kessel Run could have actually worked.

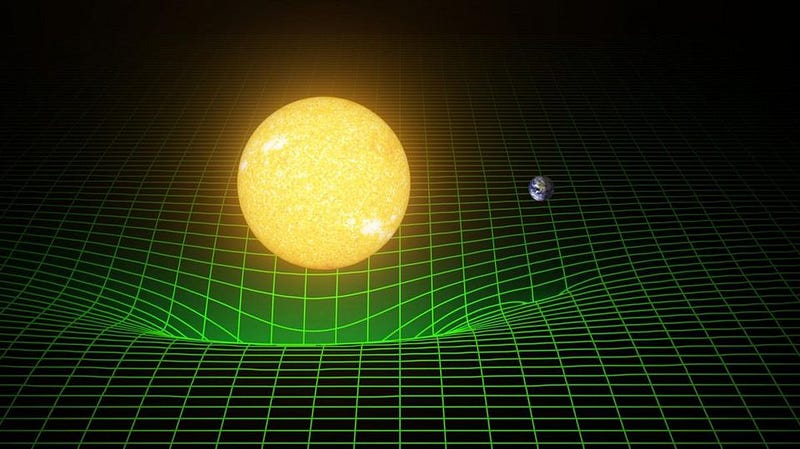
Imagine you want to navigate between any two points in space: two planets, two outposts, even two imaginary locations on a grid. You’d think, typically, that to get from one point to the other, all you’d want to do is fire your engines as fast as possible in the direction of the second point, and that’s the shortest distance (and the fastest time) between them. But this thinking is over a century out of date, since space is only perfectly flat if there are no masses in it. Place down a mass anywhere at all, and your space curves in response. That’s the cardinal rule of General Relativity: matter and energy tell spacetime how to curve; curved spacetime tells matter and energy how to move.

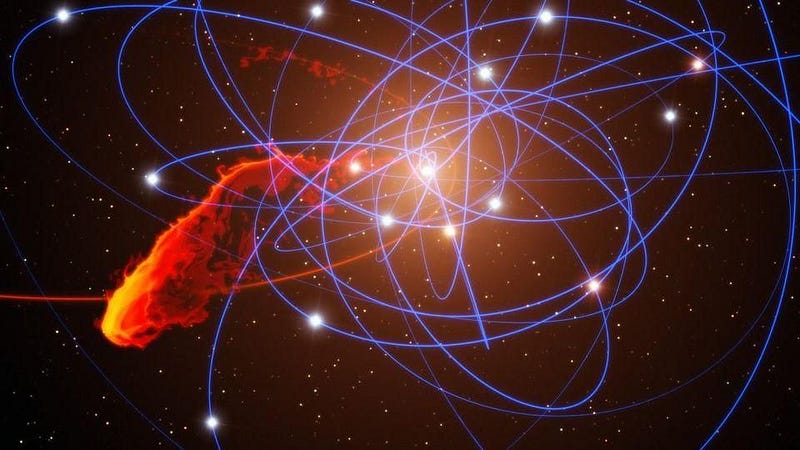
In most realistic locations in space, masses are far apart, relatively isolated, and relatively low in magnitude. In our Solar System, for example, the greatest amount of spacetime curvature is generated by our Sun, and it truly does barely curve spacetime at all. When a distant photon passes by the very edge of the Sun, the closest you can get without actually running into the Sun itself, its trajectory is deflected by less than 2″: 1/1800th of a degree. But in the vicinity of a black hole, the deflection is far more severe. Perhaps counterintuitively, it’s the lowest-mass black holes that curve space by the greatest amounts close to their event horizons.

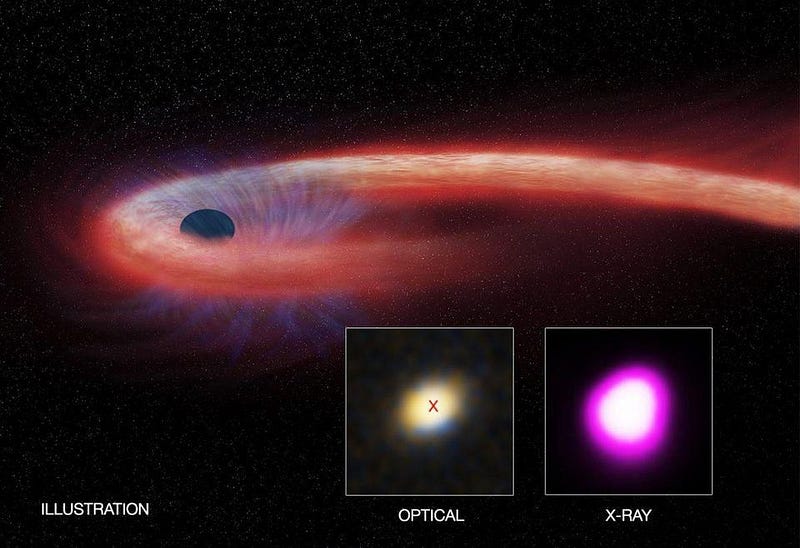
So if you want to take a shortcut through space — short of using a wormhole — your best bet is to navigate through a region of space that has very large numbers (and densities) of low-mass black holes. Surprisingly, we already know of an environment exactly like this: the galactic center. There may be thousands or even tens of thousands of low-mass black holes in the central few light years of the Milky Way, and that’s not even considering the supermassive behemoth at our galaxy’s core. The galactic center is extremely rich in matter as well, as it’s one of the dustiest, gas-rich environments known in all of space. This isn’t something that’s unique to our galaxy, but is expected to be at play in practically all spiral galaxies similar to our own.

When you travel via hyperdrive, presumably, you can’t maneuver so well. Lateral accelerations must be difficult when you’re using your faster-than-light technology, so the standard practice might be to avoid dangerous environments that are populated with potential matter debris. After all, colliding with even a small object at extremely large speeds can be catastrophic; micrometeoroids routinely blast holes in every material we’ve ever sent up into space, and those are traveling well below the speed of light.

To move quickly between two points in space, then, even a straight line might be a disastrous plan. If what you need to do is avoid a large number of potentially hazardous objects, going around might be the only option. This could mean adding a very large distance to your expected path length, perhaps adding many light years to your journey. A straight-line path might be much shorter, but much more dangerous. But the shortest path of all won’t be a straight line, but an intricately curved path through the densest, most dangerous environment of all: a field of stars, planets, black holes, gas, dust, and more. To make the Kessel Run, the Millennium Falcon may have had to go through the center of that legendary galaxy far, far away.

This would explain why the details have gotten garbled, depending on who’s telling the story. In The Force Awakens, Rey sees this large, slow vessel and asks in disbelief, “This is the ship that made the Kessel Run in fourteen parsecs?” Even the larger figure seems impossible, as maneuverability and size/mass are pretty much always inversely correlated. And yet, Han Solo corrects her, insisting, “Twelve!”

Perhaps 12 parsecs really is the shortest distance possible between the two points of the Kessel Run. And perhaps the Millennium Falcon — arguably and speculatively with a better pilot than Captain Solo — really did make the run in that shortest-possible distance. But if it did, it likely didn’t take even a straight-line path, but used that mysterious force that so few people truly understand. No, not that force that the Jedis use, but the gravitational force put forth by Einstein over 100 years ago: General Relativity. Only by taking the optimal path, through curved space, would the Millennium Falcon’s claim-to-fame be truly possible.