Ask Ethan: Why Doesn’t Gravity Happen Instantly?

It doesn’t propagate at infinite speeds, and that’s a problem for Newton.
When you look at the Sun, the light you’re seeing isn’t the light that’s being emitted right now. Instead, you’re seeing light that’s a little more than eight minutes old, since the Sun is some 150 million kilometers (93 million miles) away, and light — although it’s fast — can only travel through the Universe at a specific speed: the speed of light. But what about gravitation? Everything on Earth experiences the Sun’s gravitational pull, but is the gravity that the Earth experiences as it orbits the Sun coming from the Sun right now, at this very instant? Or, just like light, are we experiencing gravitation from some time ago? It’s a fascinating question to ponder, with Paul Roland writing in to ask about,
“the relationship of gravitational wave speed to that of light… At first I saw no connection, since gravity derives from mass and is a totally separate effect versus electromagnetics. One might assume [this] would cause gravitational effects to be slower than light [in terms of] propagation time.”
We all have our intuitive thoughts for how we expect things to behave, but only experiments and observations can provide the answer. Gravity isn’t instant, and turns out to propagate at exactly the speed of light. Here’s how we know.
Our story starts with the speed of light. The first person to try to measure it, at least according to legend, was Galileo. He set up an experiment at night, where two people would each be atop adjacent mountain peaks, each one equipped with a lantern. One of them would unveil their lantern, and when the other saw it, they would unveil their own lantern, allowing the first person to measure how much time elapsed. Unfortunately for Galileo, the results appeared instantaneous, limited only by the speed of a human’s reaction.
The key advance didn’t come until 1676, when Ole Rømer had the brilliant idea to observe Jupiter’s innermost large moon, Io, as it passed behind Jupiter and re-emerged from the giant planet’s shadow. Because light has to travel from the Sun to Io, and then from Io back to our eyes, there ought to be a delay from when Io leaves Jupiter’s shadow, geometrically, until we can observe it here on Earth. Although Rømer’s conclusions were off by about 30% from the actual value, this was the first measurement of the speed of light, and the first robust demonstration that light traveled at a finite speed after all.

Rømer’s work influenced a number of important scientists of his day, including Christiaan Huygens and Isaac Newton, who came up with the first scientific descriptions of light. About a decade after Rømer, however, Newton turned his attention to gravitation, and all ideas about a finite speed for gravity went out the window. Instead, according to Newton, every massive object in the Universe exerted an attractive force on every other massive object in the Universe, and that interaction was instantaneous.
The strength of the gravitational force is always proportional to each of the masses multiplied together, and inversely proportional to the square of the distance between them. Move twice as far away from one another, and the gravitational force becomes just one-quarter as strong. And if you ask which direction the gravitational force points in, it’s always along a straight line connecting those two masses. That’s the way Newton formulated his law of universal gravitation, where the mathematical orbits he derived matched up precisely with the way the planets moved through space.
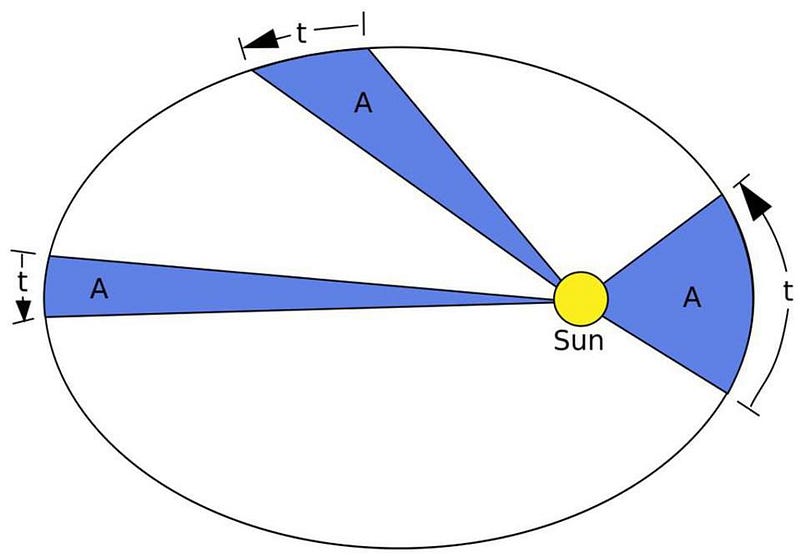
Of course, we already knew how to describe the way that planets orbited the Sun: Kepler’s laws of planetary motion were many decades old by time Newton came along. What he did that was so remarkable was to put forth a theory of gravity: a mathematical framework that obeyed rules from which all of Kepler’s laws (and many other rules) could be derived. So long as, at every moment in time, the force on any planet always points directly towards where the Sun is at that exact moment, you get the planetary orbits to match up with what we observe.
What Newton also realized was this: if you make the gravitational force point towards where the Sun was a certain amount of time ago — such as ~8 minutes ago from the perspective of planet Earth — the planetary orbits you get are all wrong. In order for Newton’s conception of gravity to have a chance at working, the gravitational force needs to be instantaneous. If gravitation is slow, even if “slow” means that it moves at the speed of light, Newton’s gravity doesn’t work, after all.
For hundreds of years, Newton’s gravity was able to solve every mechanical problem that nature (and humans) threw at it. When Uranus’s orbit appeared to violate Kepler’s laws, it was a tantalizing clue that perhaps Newton was wrong, but it wasn’t to be. Instead, there was an additional mass out there in the form of the planet Neptune. Once its position and mass became known, that puzzle went away.
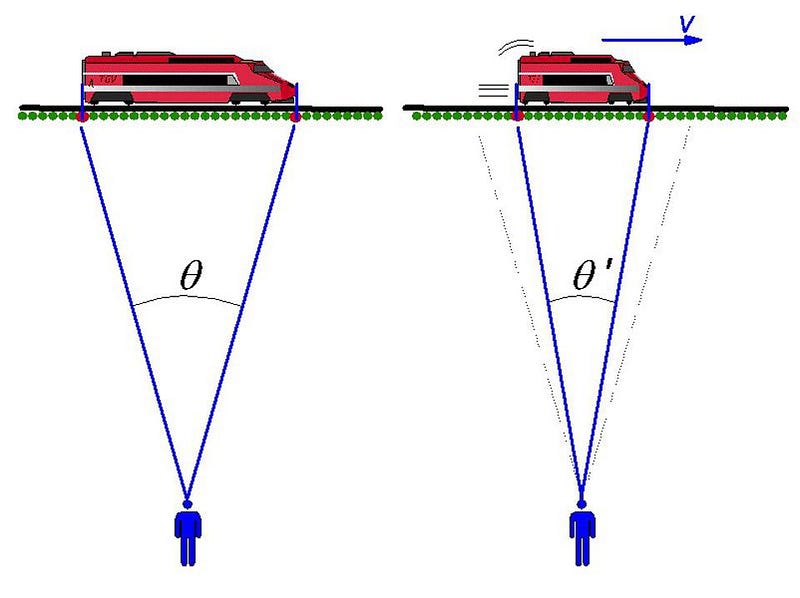
But Newton’s successes wouldn’t last forever. The first real clue came with the discovery of Special Relativity, and the notion that space and time aren’t absolute quantities, but rather how we observe them depends very intricately on our motion and location. In particular, the faster you move through space, the slower clocks appear to run and the shorter distances appear to be. As Fitzgerald and Lorentz, working before Einstein, described it, distances contract and time dilates the closer you move to the speed of light. Unstable particles are observed to survive for longer if they move at high speeds. Space and time cannot be absolute, but must be relative for each unique observer.
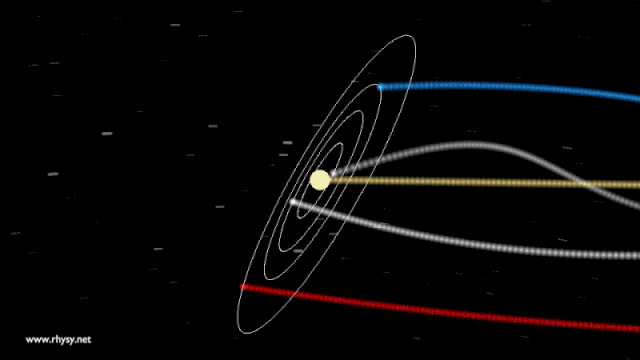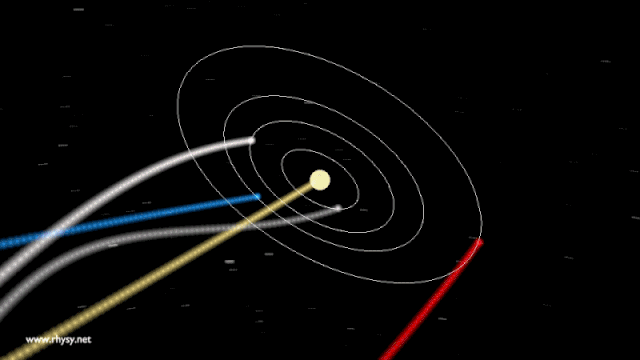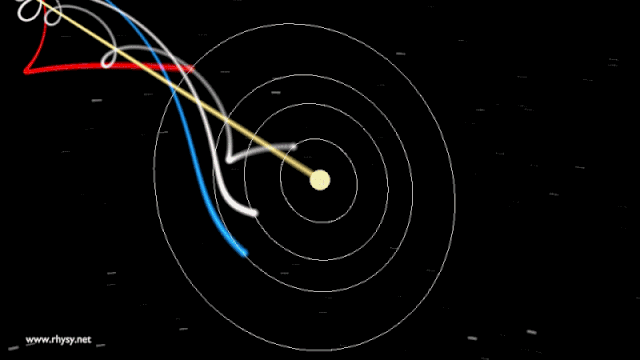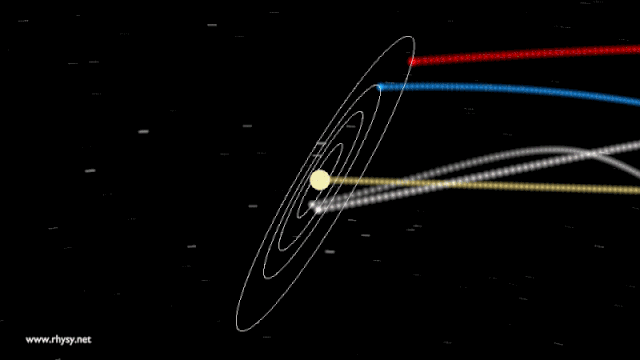
If that’s true, and different observers moving with different velocities and/or at different locations can’t agree on things like distances and times, then how could Newton’s conception of gravity be correct? It seems that all of these things can’t be true simultaneously; something must be inconsistent here.
One way to think about it is to consider an absurd but useful puzzle: imagine that, somehow, some omnipotent being were able to instantaneously remove the Sun from our Universe. What would we expect would happen to the Earth?
As far as the light goes, we know that it would continue to arrive for another 8 minutes or so, and the Sun would only appear to go disappear once that light stops reaching us. The other planets would only go dark once the sunlight stopped reaching them, reflecting off of them, and ceased arriving at our eyes. But what about gravitation? Would that cease instantly? Would all of the planets, asteroids, comets, and Kuiper belt objects simply fly off in a straight line all at once? Or would they all continue orbiting for a time, continuing their gravitational dance in blissful ignorance until the effect of gravity finally hit them?
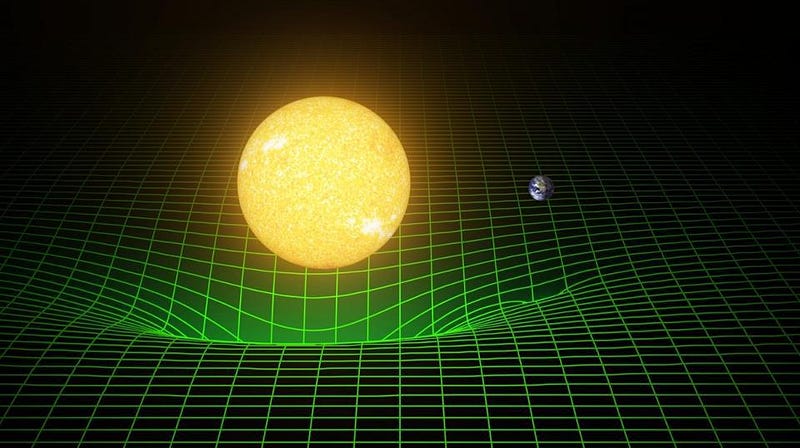
The problem, according to Einstein, is that Newton’s entire picture must be flawed. Gravity isn’t best viewed as a straight-line, instantaneous force connecting any two points in the Universe. Instead, Einstein put forth a picture where space-and-time are woven together in what he visualized as an inseparable fabric, and that not only masses, but all forms of matter and energy, deformed that fabric. Instead of the planets orbiting because of an invisible force, they simply move along the curved path determined by the curved, distorted fabric of spacetime.
This conception of gravity leads to a radically different set of equations from Newton’s, and instead predicts that gravity not only propagates at a finite speed, but that speed — the speed of gravity — must be exactly equal to the speed of light. If you were to suddenly “wink” the Sun out of existence, that spacetime fabric would “snap” back to flat the same way a rock falling into a pool of water would cause the water’s surface to snap back. It would come to equilibrium, but the changes in the surface would come in ripples or waves, and they would only propagate at a finite speed: the speed of light.

For many years, we’ve had indirect tests of the speed of gravity, but nothing that measured these ripples directly. We measured how the orbits of two pulsing neutron stars changed as they orbited one another, determining that energy was radiating away at a finite speed: the speed of light, to within a 99.8% accuracy. Just as Jupiter’s shadow obscures light, Jupiter’s gravity can bend a background light source, and a 2002 coincidence lined up Earth, Jupiter, and a distant quasar. The gravitational bending of the quasar light due to Jupiter gave us another independent measurement of gravity’s speed: it’s again the speed of light, but comes with a ~20% error.
All of this began to change dramatically about 5 years ago, when the first advanced gravitational wave detectors saw their first signals. As the first gravitational waves traveled across the Universe from merging black holes, a journey of more than a billion light-years for our first detection, they arrived at our (then) two gravitational wave detectors just milliseconds apart, a small but significant difference. Because they’re at different points on Earth, we’d expect a slightly different arrival time if gravity propagated at a finite speed, but no difference if it were instantaneous. For every gravitational wave event, the speed of light is consistent with the observed arrival times of the waves.
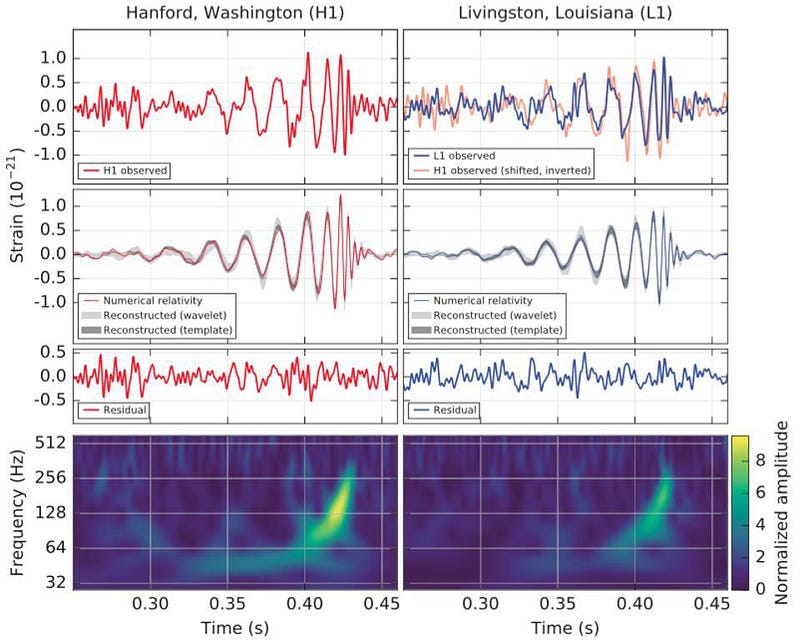
But in 2017, something spectacular happened that blew all our other constraints — both direct and indirect ones — away. From ~130 million light-years away, a gravitational wave signal began to arrive. It started out with a small but detectable amplitude, then increased in power while getting faster in frequency, corresponding to two low-mass objects, neutron stars, inspiraling and merging. After only a few seconds, the gravitational wave signal spiked, and then ceased, signaling the merger was complete. And then, no more than 2 seconds later, the first sign of light arrived: a gamma-ray burst.
It took some 130 million years for both the gravitational waves and the light from this event to travel through the Universe, and they arrived at the exact same time: to within 2 seconds. That means, at most, if the speed of light and the speed of gravity are different, then they’re different by no more than about 1 part in a quadrillion (1015), or that those two speeds are 99.9999999999999% identical. In many ways, it’s the most accurate measurement of a cosmic speed ever made. Gravity really does travel at a finite speed, and that speed is identical to the speed of light.

From a modern point of view, this makes sense, as any massless form of radiation — whether particle or wave — must travel at exactly the speed of light. What started off as an assumption based on the need for self-consistency in our theories has now been directly confirmed observationally. Newton’s original conception of gravitation doesn’t hold up, as gravity isn’t an instantaneous force after all. Instead, the results agree with Einstein: gravitation propagates at a finite speed, and the speed of gravity is exactly equal to the speed of light.
We at last know what would happen if you could somehow make the Sun disappear: the last light from the Sun would continue traveling away from it at the speed of light, and it would only go dark when the light stopped arriving. Similarly, gravity would behave in the same fashion, with the Sun’s gravitational effects continuing to influence the planets, asteroids, and all the other objects in the galaxy until its gravitational signal no longer arrived. Mercury would fly off in a straight line first, followed by all the other masses in order. The light would stop arriving at exactly the same time the gravitational effects did. As we only now know for certain, gravity and light really do travel at exactly the same speeds.
Send in your Ask Ethan questions to startswithabang at gmail dot com!
Starts With A Bang is written by Ethan Siegel, Ph.D., author of Beyond The Galaxy, and Treknology: The Science of Star Trek from Tricorders to Warp Drive.





